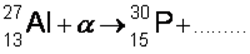Q1.

(a)

(i) Sketch a graph to show how the neutron number, N, varies with the proton number, Z, for naturally occurring stable nuclei over the range Z = 0 to Z = 90. Show values of N and Z on the axes of your graph and draw the N = Z line.

(ii) On your graph mark points, one for each, to indicate the position of an unstable nuclide which would be likely to be an emitter, labelling it A, a emitter, labelling it B. (5 marks)

(b) State the changes in N and Z which are produced in the emission of

(i) an particle,

(ii) a particle. (2 marks)

(c) The results of electron scattering experiments using different target elements show that

where A is the nucleon number and r_o is a constant. Use this equation to show that the density of a nucleus is independent of its mass. (3 marks)

(Total 10 marks)

Q2.

(a)

(i) What is meant by the random nature of radioactive decay?

(ii) Explain what is meant by each of the following:

• isotopes
• radioactive half-life
• radioactive decay constant

( 6 marks)

(b) The radioactive isotope of iodine ¹³¹I has a half-life of 8.04 days. Calculate

(i) the decay constant of ¹³¹I,

(ii) the number of atoms of ¹³¹I necessary to produce a sample with an activity of 5.0 × 10⁴ disintegrations s^–1 (Bq),

(iii) the time taken, in hours, for the activity of the same sample of ¹³¹I to fall from 5.4 × 10⁴ disintegrations s^–1 to 5.0 × 10⁴ disintegrations s^–1

(6 marks)

(Total 12 marks)

Q3.

(a) Nuclear fission can occur when a neutron is absorbed by a nucleus of uranium-235. An incomplete equation for a typical fission reaction is given below.

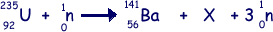
(i) State the nuclear composition of X.

(ii) Name the element of which X is an isotope. (3 marks)

(b) In a small nuclear power plant one fifth of the fission energy is converted into a useful output power of 10 MW. If the average energy released per fission is 3.2 × 10^–11J, calculate the number of uranium-235 nuclei which will undergo fission per day. (3 marks)

(Total 6 marks)

Q4.The radioisotope iodine-131 is used in medicine to treat over-active thyroid glands.

It decays into an isotope of xenon (Xe) by β^– emission with a half-life of 8.1 days.

The xenon subsequently emits a γ ray.

(a) Explain what is meant by:

(i) isotope;

(2 marks)

(ii) half-life.

(1 mark)

(b) Write down the equation which represents the nuclear reaction.

(3 marks)

(c) Calculate the time (in days) for a sample of iodine to decay to 1% of its initial activity.

(4 marks)

(d) State and explain which decay product can be detected outside the body during treatment.

(2 marks)

(Total 12 marks)

Q5.

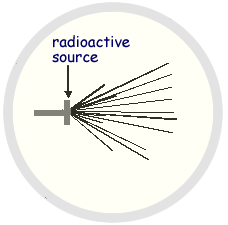(a) The diagram is copied from a photograph taken of a cloud chamber containing a small radioactive source.

(i) What type of radiation is emitted from the source?

(ii) State and explain what can be deduced about the energy of the particles emitted by the source.

(4 marks)

(b) Plutonium–239 is a radioactive isotope that emits particles of energy 5.1 MeV and decays to form a radioactive isotope of uranium. This isotope of uranium emits particles of energy 4.5 MeV to form an isotope of thorium which is also radioactive.

(i) Write down an equation to represent the decay of plutonium–239.

(ii) Write down an equation to represent the decay of the uranium isotope.

(iii) Which of the two radioactive isotopes, plutonium–239 or the uranium isotope, has the longer half-life? Give a reason for your answer.

(iv) Explain why thorium is likely to be a emitter. (5 marks)

(Total 9 marks)

Q6. A radioactive nuclide decays by emitting particles. The graph shows how the rate of decay A_t of the source changes with time t

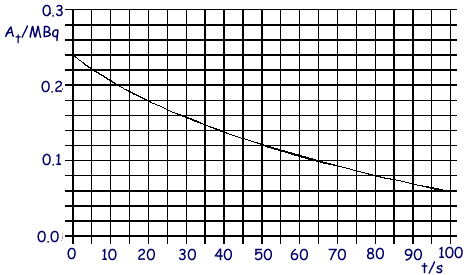
(a) Determine

(i) the half-life of the nuclide,

(ii) the decay constant,

(iii) the initial number of undecayed nuclei present at time t = 0.

(5 marks)

(b) Each decay releases 1.0 x 10^–12J.

For the time interval between t = 30s and t = 80s, calculate:

(i) the number of nuclei which decay,

(ii) the energy released.

(4 marks)

(Total 9 marks)

Q7.

(a) Sketch a graph to show how the number of neutrons, N, varies with the number of protons, Z, for stable nuclei over the range Z = 0 to Z = 80. Draw a scale on the N axis. (2)

(b) On the same graph, enclosing each region by a line, indicate the region in which nuclides are likely to decay, by

(i) emission, labelling the region A,

(ii) emission, labelling the region B,

(iii) emission, labelling the region C.

(3 marks)

(c) Complete the table.

mode of decay	change in proton number Z	change in neutron number N
emission	–2
emission
emission
e- capture
p emission		0
n emission	0

(3 marks)

(Total 6 marks)

Q8. The radioactive isotope of sodium has a half life of 2.6 years.

A particular sample of this isotope has an initial activity of 5.5 × 10⁵ Bq (disintegrations per second).

(a) Explain what is meant by the random nature of radioactive decay.

(2 marks)

(b) On the axes below, sketch a graph of the activity of the sample of sodium over a period of 6 years.

(2 marks)

(c) Calculate

(i) the decay constant, in s^–1, of ,

1 year = 3.15 × 10⁷ s

(ii) the number of atoms of in the sample initially,

(iii) the time taken, in s, for the activity of the sample to fall from 1.0 × 10⁵ Bq to 0.75 × 10⁵ Bq.

(6 marks)

(Total 10 marks)

Q9.

(a) A radioactive source gives an initial count rate of 110 counts per second. After 10 minutes the count rate is 84 counts per second.

(background radiation = 3 counts per second)

(i) Give three origins of the radiation that contributes to this background radiation.

(ii) Calculate the decay constant of the radioactive source in s^–1.

(iii) Calculate the number of radioactive nuclei in the initial sample assuming that the detector counts all the radiation emitted from the source.

(7 marks)

(b) Discuss the dangers of exposing the human body to a source of radiation. In particular compare the dangers when the source is held outside, but in contact with the body, with those when the source is placed inside the body.

(3 marks)

(Total 10 marks)

Q10.

(a) Sketch, using the axes provided, a graph of neutron number, N, against proton number, Z, for stable nuclei over the range Z = 0 to Z = 80. Show suitable numerical values on the N axis.

(2 marks)

(b) On the graph indicate, for each of the following, a possible position of a nuclide that may decay by

(i) emission, labelling the position with W,

(ii) emission, labelling the position with X,

(iii) emission, labelling the position with Y.

(3 marks)

(c) The isotope decays sequentially by emitting particles and particles, eventually forming the isotope . Four particles are emitted in the sequence. Calculate the number of particles in the sequence.

(2 marks)

(d) A particular nuclide is described as proton-rich. Discuss two ways in which the nuclide may decay.

(3 marks)

(Total 10 marks)

Q11.

(a) Suggest two reasons why an particle causes more ionization than a particle of the same initial kinetic energy.

(2 marks)

(b) A radioactive source has an activity of 3.2 × 10⁹ Bq and emits particles, each with kinetic energy of 5.2 Me V. The source is enclosed in a small aluminium container of mass 2.0 × 10^–4 kg which absorbs the radiation completely.

(i) Calculate the energy, in J, absorbed from the source each second by the aluminium container.

(ii) Estimate the temperature rise of the aluminium container in 1 minute, assuming no energy is lost from the aluminium.

specific heat capacity of aluminium = 900 J kg^–1 K^–1

(5 marks)

(Total 7 marks)

Q12. The isotope of uranium, , decays into a stable isotope of lead, by means of a series of α and β– decays.

(a) In this series of decays, α decay occurs 8 times and β– decay occurs n times. Calculate n.

(1 mark)

 

(b)

(i) Explain what is meant by the binding energy of a nucleus.

(2 marks)

(ii) The graph belowshows the binding energy per nucleon for some stable nuclides.

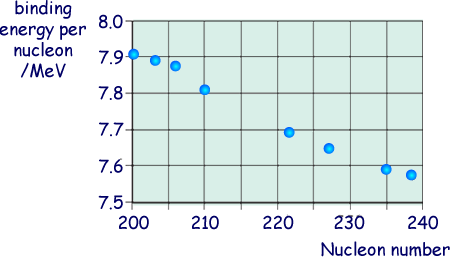
Estimate the binding energy, in MeV, of the nucleus.

(1 mark)

(c) The half-life of is 4.5 × 10⁹ years, which is much larger than all the other half-lives of the decays in the series. A rock sample when formed originally contained 3.0 × 10²² atoms of and no atoms.

At any given time most of the atoms are either or with a negligible number of atoms in other forms in the decay series.

(i) Using the axes below, sketch graphs to show how the number of atoms and the number of atoms in the rock sample vary over a period of 1.0 × 10¹⁰ years from its formation.

Label your graphs U and Pb.

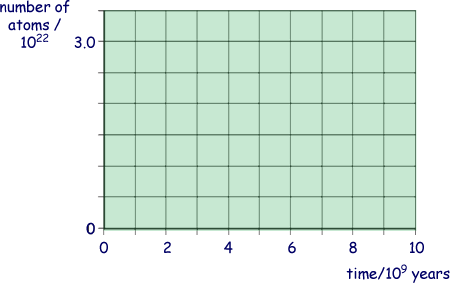
(2 marks)

(ii) A certain time, t, after its formation the sample contained twice as many atoms as atoms.

Show that the number of atoms in the rock sample at time t was 2.0 × 10²².

(1 mark)

(iii) Calculate t in years.

(3 marks)

(Total 10 marks)

Q13.

(a) An unstable nucleus, , can decay by emitting a β^– particle.

(i) What part of the atom is the same as a β^– particle?

(1 mark)

(ii) State the changes, if any, in A and Z when X decays.

(2 marks)

(b) In the process of β^– decay an anti-neutrino is also released.

(i) Give an equation for this decay.

(1 mark)

(ii) State and explain which conservation law may be used to show that it is an anti-neutrino rather than a neutrino that is released.

(2 marks)

(iii) What must be done to validate the predictions of an unconfirmed scientific theory?

(2 marks)

(Total 8 marks)

Q14.

The diagram below shows a grid of neutron number against proton number. A nucleus is marked in place:

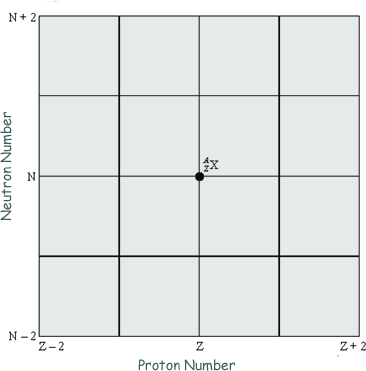
(a) Draw arrows on the grid, each starting on X and ending on a daughter nucleus after the following transitions:

(i) β^– emission (label this arrow A), neutron emission (label this arrow B), electron capture (label this arrow C).

(ii) Give the equation for electron capture by the nucleus X.

(4 marks)

(b) When decays to by β^– decay, the daughter nucleus is produced in one of two possible excited states. These two states are shown in below together with their corresponding energies.

(i) Calculate the maximum possible kinetic energy, in J, which an emitted β– particle can have.

(ii) The excited aluminium nuclei emit γ-photons. Calculate each of the three possible  γ-photon energies

(iii) Calculate the frequency of the most energetic photon emitted.

(3 marks)

(c)

(i) State and explain two precautions that should be taken when working with a sample of in a school laboratory.

(ii) Discuss which of the two types of radiation, β^– or γ, emitted from a sample of would be the more hazardous.

(3 marks)

(Total 10 marks)

Q15.

(a) Calculate the radius of the nucleus.

r₀ = 1.3 × 10^–15 m

(2 marks)

(b) At a distance of 30 mm from a point source of γ rays the corrected count rate is C.

Calculate the distance from the source at which the corrected count rate is 0.10 C, assuming that there is no absorption.

(2 marks)

(c) The activity of a source of β particles falls to 85% of its initial value in 52 s.

Calculate the decay constant of the source.

(3 marks)

(d) Explain why the isotope of technetium, Tc 99m, is often chosen as a suitable source of radiation for use in medical diagnosis.

(3 marks)

(Total 10 marks)

Q16.

(a) The lead nuclide is unstable and decays in three stages through α and β emissions to a different lead nuclide . The position of these lead nuclides on a grid of neutron number, N, against proton number, Z, is shown on the grid below:

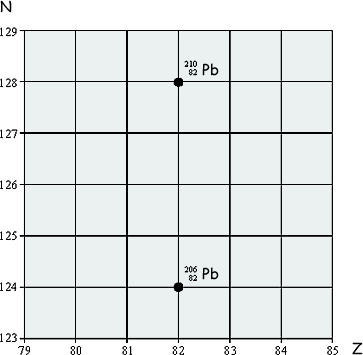
On the grid draw three arrows to represent one possible decay route.

Label each arrow with the decay taking place.

(3 marks)

(b) The copper nuclide 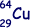may decay by positron emission or by electron capture to form a nickel (chemical symbol - Ni) nuclide. Write the two equations that represent these two possible modes of decay.

(4 marks)

(c) The nucleus of an atom may be investigated by scattering experiments in which radiation or particles bombard the nucleus.

Name one type of radiation or particle that may be used in this investigation and describe the main physical principle of the scattering process.

State the information which can be obtained from the results of this scattering.

(3 marks)

(Total 10 marks)

Q17. The first artificially produced isotope, phosphorus-30, was formed by bombarding an aluminium-27 isotope,with an α particle.

(a) Complete the following nuclear equation by identifying the missing particle.

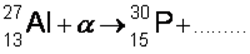
(1 mark)

(b) For the reaction to take place the α particle must come within a distance, d, from the centre of the aluminium nucleus.

Calculate 'd' if the nuclear reaction occurs when the α particle is given an initial kinetic energy of at least 2.18 × 10^–12 J.

The electrostatic potential energy between two point charges Q₁ and Q₂ is equal to:

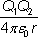
where

r is the separation of the charges and

ε₀ is the permittivity of free space.

(3 marks)

(Total 4 marks)

Q18.

(a) Which of the following forms of ionizing radiation produces the greatest number of ion pairs per mm in air? (Tick the correct answer)

α particles
β particles
γ rays
X-rays

[1 mark]

(b)

(i) Complete the table below showing the typical maximum range in air for α and β particles.

Radiation Type	Typical maximum range in air /m
α
β

 

[2 marks]

(ii) γ rays have a range of at least 1 km in air.

However, a γ ray detector placed 0.5 m from a γ ray source detects a noticeably smaller count-rate as it is moved a few centimetres further away from the source.

Explain this observation.

[1 mark]

(c) Following an accident, a room is contaminated with dust containing americium (which is an α-emitter). Explain the most hazardous aspect of the presence of this dust to an unprotected human entering the room.

[2 marks]

(Total 6 marks)

You may also want to try Carbon Dating Questions

or questions relating to practical experiments with radioactive isotopes.