Solutions: Radioactivity Questions
Q4.The radioisotope iodine-131 
is used in medicine to treat over-active thyroid glands.
It decays into an isotope of xenon (Xe) by β– emission with a half-life of 8.1 days.
The xenon subsequently emits a γ ray.
(a) Explain what is meant by:
(i) isotope;
Isotopes are nuclides (or atoms) of the same element. They have the same number of protons 
in the nucleus but a different number of neutrons.
(2 marks)
(ii) half-life.
The half life of a radioactive nuclide is the time taken for half ofthe number of nuclei of that nuclide (not just 'atoms') in the sample to decay.
or
The half life of a radioactive nuclide is the time taken for the activity of the sample to fall to fall to half its initial value.
(1 mark)
(b) Write down the equation which represents the nuclear reaction.
(3 marks)
(c) Calculate the time (in days) for a sample of iodine to decay to 1% of its initial activity.
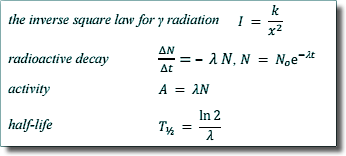
Thalf = ln 2/λ
λ = ln 2/Thalf
λ = ln 2/8.1 days-1
λ = 0.0856 days-1 |
N/No = A/Ao = 0.01
N = No e-λt
0.01 = e-λt
ln 0.01 = -λt
t = (ln 0.01)/0.0856 days
t = 53.8 days
time = 54 days
|
(4 marks)
(d) State and explain which decay product can be detected outside the body during treatment.
The γ radiation detected outside the body as its penetration power is great enough to penetrate tissue, The β– cannot penetrate tissue. It would be absorbed by the body.
(2 marks)
(Total 12 marks)
About this site
