Q1. A single gas molecule of mass m is moving in a rectangular box with a velocity of u_x in the positive x-direction, as shown in the diagram. The molecule moves backwards and forwards in the box, striking the end faces normally and making elastic collisions.

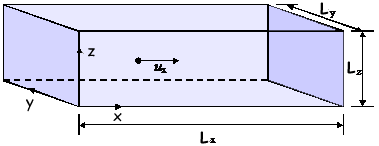
(a)

(i) Show that the time, t, between collisions with the shaded face, is:

t = 2L_x/u_x

(ii) In terms of m and u_x write down an expression for the change of momentum per collision with the shaded face.

(2 marks)

(b) If it is assumed that the box contains N identical molecules, each of mass m, all moving parallel to the x-direction with speed u_x and making elastic collisions at the ends, show that the average force, F, on the shaded face is given by

F = Nmu_x²/L_x

(2 marks)

(c) In a better model of molecular motion in gases, molecules of mean square speed are assumed to move randomly in the box.

(i) By considering this random motion obtain an expression for u_x²in terms of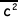

(ii) Show that a better expression for F is

F = Nm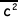/(3L_x)

(iii) Hence derive the equation pV = ¹/₃ Nm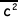

(5 marks)

(Total 9 marks)

Q2.

(a) Use the kinetic theory of gases to explain why:

(i) the pressure exerted by an ideal gas increases when it is heated at constant volume,

(ii) the volume occupied by an ideal gas increases when it is heated at constant pressure.

(4 marks)

(b) A quantity of 0.25 mol of air enters a diesel engine at a pressure of 1.05 × 10⁵ Pa and a temperature of 27°C. Assume the gas to be ideal.

(i) Calculate the volume occupied by the gas.

(ii) When the gas is compressed to one twentieth of its original volume the pressure rises to 7.0 × 10⁶ Pa. Calculate the temperature of the gas immediately after the compression.

(4 marks)

(Total 8 marks)

Q3.

(a) State two quantities which increase when the temperature of a given mass of gas is increased at constant volume.

(2 marks)

(b) A car tyre of volume 1.0 × 10^–2 m³ contains air at a pressure of 300 kPa and a temperature of 290 K. The mass of one mole of air is 2.9 × 10^–2 kg. Assuming that the air behaves as an ideal gas, calculate

(i) n, the amount, in mol, of air,

(ii) the mass of the air,

(iii) the density of the air.

(5 marks)

(c) Air contains oxygen and nitrogen molecules. State, with a reason, whether the following are the same for oxygen and nitrogen molecules in air at a given temperature.

(i) The average kinetic energy per molecule

(ii) The r.m.s. speed

(4 marks)

(Total 11 marks)

Q4.

(a) The molecular theory model of an ideal gas leads to the derivation of the equation

pV = ¹/₃ Nm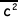

Explain what each symbol in the equation represents.

(4 marks)

(b) One assumption used in the derivation of the equation stated in part (a) is that molecules are in state of random motion.

(i) Explain what is meant by random motion.

(ii) State two more assumptions used in this derivation.

(3 marks)

(c) Describe how the motion of gas molecules can be used to explain the pressure exerted by a gas on the walls of its container.

(4 marks)

(Total 11 marks)

Q5.

(a) The volume of air in a fully expanded pair of human lungs is 5.0 × 10^–3 m³. The pressure of the air in the lungs is 1.0 × 10⁵ Pa and its temperature is 310 K.

Calculate:

(i) the number of moles of air in the lungs,

(ii) the average kinetic energy of an air molecule in the lungs.

(4 marks)

(b) Air is a mixture of oxygen and nitrogen molecules. The mass of an oxygen molecule is greater than the mass of a nitrogen molecule. State and explain the effect this has on the mean square speeds of the oxygen and nitrogen molecules in the lungs.

(3 marks)

(Total 7 marks)

Q6.

The table gives the average kinetic energy of gas molecules at certain temperatures.

Ek/J × 10–21	6.21	6.62	7.04	7.45	7.87	8.28
T/K	300	320	340	360	380	400

 

(a) On a sheet of A4 graph paper plot a graph of Ek against T.

(i) Use your graph to determine the average kinetic energy of gas molecules at 350 K.

(ii) Determine the gradient of your graph and hence calculate a value for the Boltzmann constant. Show all your working.

(8 marks)

(b) One of the assumptions of the kinetic theory is that collisions of gas molecules are elastic.

(i) State what is meant by an elastic collision.

(ii) State another assumption of the kinetic theory.

(iii) Explain how the data in the table leads to the concept of absolute zero.

(4 marks)

(Total 12 marks)

Q7.

The number of molecules in one cubic metre of air decreases as altitude increases. The table shows how the pressure and temperature of air compare at sea-level and at an altitude of 10 000 m.

altitude	pressure/Pa	temperature/K
sea-level	1.0 × 105	300
10 000 m	2.2 × 104	270

 

(a) Calculate the number of moles of air in a cubic metre of air at:

(i) sea-level,

(ii) 10 000 m.

(3 marks)

(b) In air, 23% of the molecules are oxygen molecules. Calculate the number of extra oxygen molecules there are per cubic metre at sea-level compared with a cubic metre of air at an altitude of 10 000 m.

(2 marks)

(Total 5 marks)

Q8.

(a) A cylinder of fixed volume contains 15 mol of an ideal gas at a pressure of 500 kPa and a temperature of 290 K.

(i) Show that the volume of the cylinder is 7.2 × 10^–2 m³.

(ii) Calculate the average kinetic energy of a gas molecule in the cylinder.

(4 marks)

(b) A quantity of gas is removed from the cylinder and the pressure of the remaining gas falls to 420 kPa. If the temperature of the gas is unchanged, calculate the amount, in mol, of gas remaining in the cylinder.

(2 marks)

(c) Explain in terms of the kinetic theory why the pressure of the gas in the cylinder falls when gas is removed from the cylinder.

(4 marks)

(Total 10 marks)

Q9.

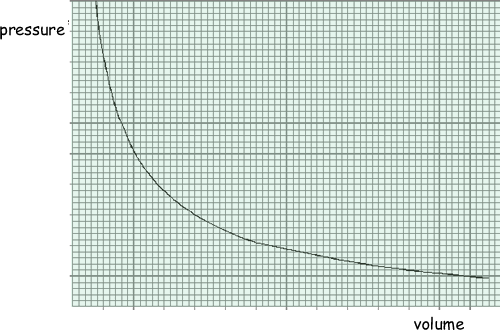
The graph shows how the pressure of an ideal gas varies with its volume when the mass and temperature of the gas are constant.

(a) On the same axes, sketch two additional curves A and B, if the following changes are made.

(i) The same mass of gas at a lower constant temperature (label this A).

(ii) A greater mass of gas at the original constant temperature (label this B).

(2 marks)

(b) A cylinder of volume 0.20 m³ contains an ideal gas at a pressure of 130 kPa and a temperature of 290 K. Calculate

(i) the amount of gas, in moles, in the cylinder,

(ii) the average kinetic energy of a molecule of gas in the cylinder,

(iii) the average kinetic energy of the molecules in the cylinder.

(5 marks)

(Total 7 marks)

Q10.

(a)

(i) Write down the equation of state for n moles of an ideal gas.

(ii) The molecular kinetic theory leads to the derivation of the equation pV = ¹/₃ Nm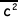 , where the symbols have their usual meaning. State three assumptions that are made in this derivation.

(4 marks)

(b) Calculate the average kinetic energy of a gas molecule of an ideal gas at a temperature of 20 °C.

(3 marks)

(c) Two different gases at the same temperature have molecules with different mean square speeds. Explain why this is possible.

(2 marks)

(Total 9 marks)

Q11.

(a)

(i) One of the assumptions of the kinetic theory of gases is that molecules make elastic collisions. State what is meant by an elastic collision.

(ii) State two more assumptions that are made in the kinetic theory of gases.

(3 marks)

(b) One mole of hydrogen at a temperature of 420 K is mixed with one mole of oxygen at 320 K. After a short period of time the mixture is in thermal equilibrium.

(i) Explain what happens as the two gases approach and then reach thermal equilibrium.

(ii) Calculate the average kinetic energy of the hydrogen molecules before they are mixed with the oxygen molecules.

(4 marks)

(Total 7 marks)

Q12.

(a) State the equation of state for an ideal gas.

(1 mark)

(b) A fixed mass of an ideal gas is heated while its volume is kept constant. Sketch a graph on the axes below to show how the pressure, p, of the gas varies with the absolute temperature, T, of the gas.

(2 marks)

(c) Explain in terms of molecular motion, why the pressure of the gas in part (b) varies with the absolute temperature.

(4 marks)

(d) Calculate the average kinetic energy of the gas molecules at a temperature of 300 K.

(2 marks)

(Total 9 marks)

Q13.

(a) The air in a room of volume 27.0 m³ is at a temperature of 22 °C and a pressure of 105 kPa. Calculate

(i) the temperature, in K, of the air,

(ii) the number of moles of air in the room,

(iii) the number of gas molecules in the room.

(5 marks)

(b) The temperature of an ideal gas in a sealed container falls. State, with a reason, what happens to the

(i) mean square speed of the gas molecules,

(ii) pressure of the gas.

(4 marks)

(Total 9 marks)

Q14. The pressure inside a bicycle tyre of volume 1.90 × 10^–3m³ is 3.20 × 10⁵ Pa when the temperature is 285 K.

(a)

(i) Calculate the number of moles of air in the tyre.

(1 mark)

(ii) After the bicycle has been ridden the temperature of the air in the tyre is 295 K. Calculate the new pressure in the tyre assuming the volume is unchanged. Give your answer to an appropriate number of significant figures.

(3 marks)

(b) Describe one way in which the motion of the molecules of air inside the bicycle tyre is similar and one way in which it is different at the two temperatures.

(2 marks)

(Total 6 marks)

Q15. The diagram shows an arrangement that is used to measure the density of a powder.

The air in the cylinder is forced into the flask and the air pressure is measured, by the pressure gauge P, before and after the change. The test is then repeated with the powder present in the flask.

In both tests, the initial pressure in the flask is the same.

(a)

(i) Explain why, after compression of the air the pressure in the flask is greater when the powder is present than when it is not present.

(ii) Calculate the pressure in the flask, after compression at constant temperature, when no powder was present. Assume that the volume of the tubes and pressure gauge is negligible.

volume of the empty flask = 2.50 × 10^–4 m³

volume of the cylinder = 1.00 × 10^–4 m³

initial pressure of the air in the flask and cylinder = 100 kPa.

(6 marks)

(b) To test the apparatus, 0.13 kg of powder of density 2700 kg m^–3 was placed in the flask before compression.

(i) Calculate the volume of this amount of powder.

(ii) The pressure of the air in the flask increased to 150 kPa when the test was carried out with this amount of powder in the flask. By carrying out an appropriate calculation, justify whether or not the test was successful

(5 marks)

(Total 11 marks)

Q16.

(a) The concept of an absolute zero of temperature may be explained by reference to the behaviour of a gas. Discuss one experiment that can be performed using a gas which would enable you to explain absolute zero and determine its value.

It is not necessary to give full details of the apparatus.

Your answer should:

include the quantities that are kept constant

identify the measurements to be taken

explain how the results may be used to find absolute zero

justify why the value obtained is absolute zero.

[6 marks]

(b)

(i) State two assumptions about the movement of molecules that are used when deriving the equation of state, pV = – ¹/₃ N m (c_^rms)² for an ideal gas.

[2 marks]

(ii) Three molecules move at the speeds shown in the table below:

molecule	speed / m s–1
1	2000
2	3000
3	7000

 

Calculate their mean square speed.

[3 marks]

(c) The average molecular kinetic energy of an ideal gas is 6.6 × 10^–21 J.

Calculate the temperature of the gas.

[3 marks]

(14 marks total)