A level: Kinetic Theory Questions
Q8.
(a) A cylinder of fixed volume contains 15 mol of an ideal gas at a pressure of 500 kPa and a temperature of 290 K.
(i) Show that the volume of the cylinder is 7.2 × 10–2 m3.
pV = nRT
V = nRT/p
= 15 x 8.31 x 290/(500 x 103) 
= 7.23 x 10-2 m2
(ii) Calculate the average kinetic energy of a gas molecule in the cylinder.
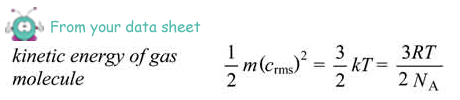
Ek = 3/2 kT
= 3/2 x 1.38 x 10-23 x 290
= 6.0 × 10–21 J
(4 marks)
(b) A quantity of gas is removed from the cylinder and the pressure of the remaining gas falls to 420 kPa. If the temperature of the gas is unchanged, calculate the amount, in mol, of gas remaining in the cylinder.
pV = nRT
n = pV/RT = (420 x 103 x 7.2 × 10–2)/(8.31 x 290)
= 13 mol
p1/n1 = p2/n2
n2 =p2n1/p1
n2 = 420 x 15/500
= 13 mol (to 2 sig figs)
(2 marks)
(c) Explain in terms of the kinetic theory why the pressure of the gas in the cylinder falls when gas is removed from the cylinder.
Pressure is due to molecular bombardment upon the container walls. Although the mean square speed of the molecules will remain constant,,when gas is removed there are fewer molecules in the cylinder [or the density of the gas decreases]. This means that the rate of bombardment of the container walls will also decrease and the force on the walls will decrease resulting in a reduction of pressure.
(4 marks maximum)
(Total 10 marks)
About this site
