A level: Kinetic Theory Questions
Q12.
(a) State the equation of state for an ideal gas.
pV = nRT 
(1 mark)
(b) A fixed mass of an ideal gas is heated while its volume is kept constant. Sketch a graph on the axes below to show how the pressure, p, of the gas varies with the absolute temperature, T, of the gas.
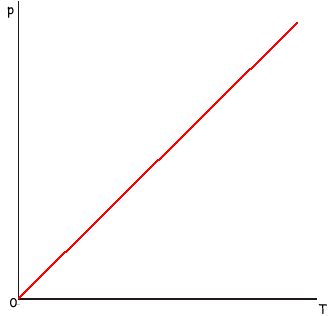
Graph to show: straight line through the origin
(2 marks)
(c) Explain in terms of molecular motion, why the pressure of the gas in part (b) varies with the absolute temperature.
The average kinetic energy or speed of the molecules increases with temperature. At higher temperatures collisions with the container walls occur more frequently[or more particles hit the walls (per second)]. The higher speed means that the (average) change in momentum (during a collision) is greater, this combined with greater frequency of collision means that the rate of change of momentum is greater and as the force produced is due to the rate of change of momentum, the force on the container (and therefore the pressure) produced is greater
(4 marks)
(d) Calculate the average kinetic energy of the gas molecules at a temperature of 300 K.
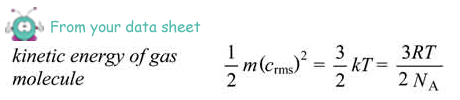
average Ek per molecule = 3/2 kT
= 1.5 × 1.38 × 10–23 × 300 = 6.2× 10–21 J
(2 marks)
(Total 9 marks)
About this site
