5.1.1
Temperature |
(a) thermal equilibrium |
|
|
| (b) absolute scale of temperature (i.e. the thermodynamic scale) that does not depend on property of any particular substance. |
|
|
(c) temperature measurements both in degrees Celsius (°C) and in kelvin (K) |
|
|
| (d) T(K) ≈ θ(°C) + 273 |
|
|
5.1.2
Solid, liquid and gas |
(a) solids, liquids and gases in terms of the spacing, ordering and motion of atoms or molecules |

|
(b) simple kinetic model for solids, liquids and gases |
(c) Brownian motion in terms of the kinetic model of matter and a simple demonstration using smoke particles suspended in air |
(d) internal energy as the sum of the random distribution of kinetic and potential energies associated with the molecules of a system |
(e) absolute zero (0 K) as the lowest limit for temperature; the temperature at which a substance has minimum internal energy |
(f) increase in the internal energy of a body as its temperature rises |
| (g) changes in the internal energy of a substance during change of phase; constant temperature during change of phase. |
5.1.3
Thermal properties of materials |
(a) specific heat capacity of a substance;
the equation
E = mcΔθ
Estimating specific heat capacity, using method of mixture. |
E = mcΔθ |
|
| (b) (i) an electrical experiment to determine the specific heat capacity of a metal or a liquid |
|
|
(b) (ii) techniques and procedures used for an electrical method to determine the specific heat capacity of a metal block and a liquid |
|
|
(c) specific latent heat of fusion and specific latent heat of vaporisation;
E = mL |
E = mL |
|
(d) (i) an electrical experiment to determine the specific latent heat of fusion and vaporisation |
|
|
(d) (ii) techniques and procedures used for an electrical method to determine the specific latent heat of a solid and a liquid. |
|
|
5.1.4
Ideal gases |
(a) amount of substance in moles; Avogadro constant NA equals 6.02 × 1023 mol–1 |
|
|
(b) model of kinetic theory of gases assumptions for the model:
- large number of molecules in random, rapid motion particles (atoms or molecules) occupy negligible volume compared to the volume of gas
- all collisions are perfectly elastic and the time of the collisions is negligible compared to the time between collisions
- negligible forces between particles except during collision |
|
|
| (c) pressure in terms of this model.
|
|
Explanation of pressure in terms of Newtonian theory. |
| (d) (i) the equation of state of an ideal gas pV = nRT, where n is the number of moles |
pV = nRT |
|
(d) (ii) techniques and procedures used to investigate
PV = constant (Boyle's law) and
T/P = constant |
|
|
(d) (iii) an estimation of absolute zero using variation of gas temperature with pressure |
|
|
(e) the equation
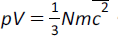
where N is the number of particles (atoms or molecules) and c2 is the mean square speed |
|
Derivation of this equation is not required.... but you might find it interesting to look at! Click here |
(f) root mean square (r.m.s.) speed; mean square speed |
|
Learners should know about the general characteristics of the Maxwell-Boltzmann distribution. |
(g) the Boltzmann constant;
|
k = R/NA |
|
(h) pV = NkT;
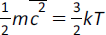
|
pV = NkT |
Learners will also be expected to know the derivation of this equation from
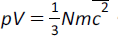
and pV = nkT
See here |
(i) internal energy of an ideal gas. |
|
|


