Topic Menu

Cyber-chess a chess instruction site

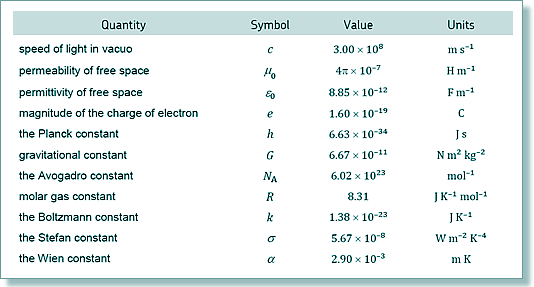
Nuclear Fission

Q1. What is the binding energy of the nucleus ?

Use the following data:

mass of a proton = 1.00728 u

mass of a neutron = 1.00867 u

mass of a U nucleus = 238.05076 u

1 u = 931.3 MeV

mass defect = mass of nucleus - mass of individual nucleons

neutron number: 238 - 92 = 146

Δm = 238.05076u - (92 x 1.00728 + 146 x 1.00867)u

Δm =-1.88482u

Δm = -1.88482 x 931.3 MeV

Δm = -1755 MeV

A
 1685 MeV
B
 1732 MeV
C
 1755 MeV
D
 1802 MeV

 

Q2. The fission of one nucleus of uranium 235 releases 200 MeV of energy. What is the value of this energy in J?

200 MeV = 200 x 106 eV = 200 x 106 x 1.6 x 10-19 J = 3.2 x 10-11 J

A
 3.2 × 10-25J
B
 3.2 × 10-17J
C
 3.2 × 10-11J
D
 2.0 × 106J

 

Q3. The mass of the nuclear fuel in a nuclear reactor decreases at a rate of 1.2 × 10–5 kg per hour. Assuming 100% efficiency in the reactor what is the power output of the reactor?

1.2 × 10–5 kg per hour = 1.2 × 10–5/602 kg per second

= 1.2 × 10–5/(1.661 x 10-27x 602) u per second

= 931.3 x 1.2 × 10–5/(1.661 x 10-27x 602) MeV per second

= 931.3 x 12/(1.661 x 10-27x 602) eV per second

= 1.6 x 10-19 x 931.3 x 12/(1.661 x 10-27x 602) J per second

= 3.0 x 108 W

= 3.0 x 102 MW

 

A
 100 MW
B
 150 MW
C
 200 MW
D
 300 MW

 

Q4. The moderator in a nuclear reactor is sometimes made of graphite. What is the purpose of the graphite?

A
to absorb all the heat produced
B
to decrease the neutron speeds
C
to absorb alpha and gamma radiations
D
to prevent the reactor from going critical

 

Q5. Which line, A to D, in the table gives a combination of materials that is commonly used for moderating, controlling and shielding respectively in a nuclear reactor?

 

moderating

controlling

shielding

A

graphite

carbon

lead

B

cadmium

carbon

concrete

C

cadmium

boron

lead

D

graphite

boron

concrete

 

Q6. Which one of the following statements is not true about the control rods used in a nuclear reactor?

A
 They must absorb neutrons.
B
They must slow down neutrons to thermal speeds.
C
They must retain their shape at high temperatures.
D
The length of rod in the reactor must be variable.

 

Q7. A thermal nuclear reactor is shut down by inserting the control rods fully into the core. Which line, A to D, shows correctly the effect of this action on the fission neutrons in the reactor?

 

number of fission neutrons

average kinetic energy of fission neutrons

A
B
C
D

reduced
reduced
unchanged
unchanged

reduced
unchanged
reduced
unchanged

 

Q8. For a nuclear reactor in which the fission rate is constant, which one of the following statements is correct?

A
 There is a critical mass of fuel in the reactor.
B
 For every fission event, there is, on average, one further fission event.
C
 A single neutron is released in every fission event.
D
 No neutrons escape from the reactor.

 

Q9. Artificial radioactive nuclides are manufactured by placing naturally-occurring nuclides in a nuclear reactor. They are made radioactive in the reactor as a consequence of bombardment by

A
 alpha particles.
B
 beta particles
C
 protons
D
 neutrons

 

Q10. In a thermal reactor, induced fission is caused by the nucleus capturing a neutron, undergoing fission and producing more neutrons. Which one of the following statements is true?

A
To sustain the reaction a large number of neutrons is required per fission.
B
 The purpose of the moderator is to absorb all the heat produced.
C
The neutrons required for induced fission of U should be slow neutrons.
D
The purpose of the control rods is to slow down neutrons to thermal speeds.

 

Q11. Why is a moderator required in a thermal nuclear reactor?

A
 to prevent overheating of the nuclear core
B
 to absorb surplus uranium nuclei
C
 to shield the surroundings from gamma radiation
D
 to reduce the kinetic energy of fission neutrons

 

Q12. The nuclear fuel, which provides the power output in a nuclear reactor, decreases in mass at a rate of 6.0 × 10–6 kg per hour. What is the maximum possible power output of the reactor?

A
 42 kW
B
 75 MW
C
 150 MW
D
 300 MW

 

6.0 × 10–6 kg is equivalent to 6.0 × 10–6/1.661 × 10–27 u = 931.5 x 6.0 × 10–6/1.661 × 10–27MeV

therefore in 1 second the energy output is 931.5 x 6.0 × 10–6/(1.661 × 10–27 x 602) MeV

= 9.347 x 1020 MeV

= 9.347 x 1026 eV

energy each second = 9.347 x 1026 x 1.60 x 10-19 J

energy each second = 1.5 x 108 J

That happens each second so power output = 150 MW - choice C