Topic Menu

Cyber-chess a chess instruction site

Module 3:

Section 3.1 Motion

3.1.1

Motion along a straight line

 

 

 

 

 Displacement, instantaneous speed, average speed,

 

 

 You met speed at KS3
velocity  
and acceleration.  
Representation by graphical methods of uniform and non-uniform acceleration, displacement, speed,
  

You need to know the dynamics graphs that you met at GCSE

You have to know how to draw graphs to a high standard  - and how to find gradients and areas under graphs (differentiation and integration will not be called for)

Interpretation of velocity-time and displacement-time graphs for uniform and non-uniform acceleration; Need to understand the physical significance of areas under graph lines and gradients.

Area under a v/t graph between two times is the distance travelled in that time interval

Gradient of s/t graph is velocity and gradient of v/t graph is acceleration

3.1.2

Equations for uniform acceleration

 

 

 The equations of motion for constant acceleration in a straight line, including motion of bodies falling in a uniform gravitational field without air resistance

Called Newton's Equations of motion.

 

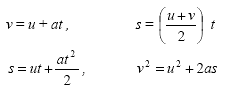
Don't forget the interactive XL Spreadsheets

Techniques and procedures used to investigate the motion and collisions of objects

Multiple Choice

Structured Questions

Apparatus may include trolleys, air-track gliders, ticker timers, light gates, data-loggers and video techniques.

This relates to momentum so I am surprised to find it here... see 3.5
Acceleration due to gravity, g of free fall;
  Techniques and procedures used to determine the acceleration of free fall using trapdoor and electromagnet arrangement or light gates and timer
Reaction time and thinking distance; braking distance and stopping distance for a vehicle.   Again relates to change in momentum... see 3.5

3.1.3

Projectile motion

 Independence of the vertical and horizontal motion of a projectile   Don't forget the interactive XL Spreadsheets
Two-dimensional motion of a projectile with constant velocity in one direction and constant acceleration in a perpendicular direction.   The constant acceleration is due to gravity and the projectile is for example a horizontally fired bullet....