Dealing with Errors when Using Graphs
Gradient of a straight line
Gradient of a curve
Intercept
Plotting graphs
Relationships involving powers
Straight line graph

The straight line graph

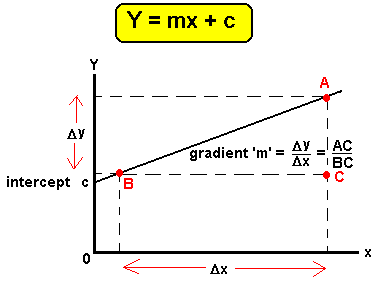
The general form of a linear equation is y = mx + c in which y is taken to be the dependent and x the independent variable; this is the one that you vary. with m and c being constants. The gradient of the line. which may be negative, gives the value of m whilst the intercept on the vertical axis gives the value of c.

A graph is used to summarise data in a pictorial way such that the main features of the relationship under investigation can be seen. Since linear relationships are easier to 'see' the straight line graph is of great importance in physics.

Plotting graphs

In order to extract the maximum of reliable data from a graph it is important to make full use of the graph paper,

Whenever it is available make use of 1mm A4 graph paper but in all case use A4 graph paper

When choosing a scale for the graph ensure your data covers at least 8cm by 8cm on that scale

When finding the gradient make sure that you use the largest possible values for Δx and Δy.

Include error bars and / or least and greatest gradient lines

Do not forget the units for m and c

The intercept

For an equation of the form y = mx + c when x = 0 then y equals c

WARNING!

If the x-axis does not start at x = 0

then the intercept on the y-axis WILL NOT give the value of c.

Sometimes in order to generate a more sensible scale it is better not to start at x = 0 but the above warning must then be remembered.

The y-axis does not, however, need to start at y = 0 in order to obtain the value of c from the intercept

The gradient

If we consider the equation y = mx + c then we can transpose to give;

m =(y-c) / x

You should also notice that the gradient is given by

                 change in gradient = change in y value  change in x value

OR

gradient = Dy/Dx

Relationships involving powers

Not all of the relationships you investigate will be linear in nature, many will be of the form y = kxⁿ where k and n are constants. Plotting a graph of y and x would produce a curve which would not allow k or n to be found. This can be resolved be the use of logs.

y = kxⁿ

log y = log k + n (log x)

or

log y= n log x + log k

this is now in the form of

y =mx + c
 
 

So, if you now plot log y on the vertical axis and log x on the horizontal a straight line will be produced which will allow 'n' to be found from the gradient and log k to be found from the intercept. This, in turn, allows k to be found.

When you plot a log graph the log values need to be tabulated; note that log values do not have units.
 

P.D. (V)	log (P.D./V)*	current (A)	log (current/A)*
20.0	1.30	0.60	- 0.22**
40.0	1.60	1.6	0.20
60.0	1.78	3.3	0.52
80.0	1.90	4.9	0.69
100	2.00	6.5	0.81

* use a sensible number of significant figures - you have got to plot the data later!

** take care - log values can be negative

The gradient of a curve

Rather than draw a log graph to turn a curve into a straight line it is sometimes useful to use a curve in order to more readily see the trend of the results.

Information is sometimes required that means finding the gradient of the curve at a given point.

This is done by drawing a tangent to the curve at that point. A tangent only touches the curve at that one point and is said to be 'perpendicular to the normal of the curve at that point'

Drawing tangents is often utilised in work involving varying rates.

Dealing with Errors when Using Graphs

When a graph of the form y = mx + c is plotted it produces a straight line. The gradient of such a graph is m and the intercept c. These values are often the quantities we are trying to find in our investigation and as such some estimation of the error in them needs to be made.

The best fit line is used to give a measurement of the gradient and intercept.

This must be shown in your coursework.

However if we wish to estimate the error then we can draw the two additional lines:

- one the steepest possible through the points and

- one at the shallowest gradient.

The gradient of the steeper of the two is called M_max and the other M_min.

The error in the value of m can be estimated as

Dm = ½ (M_max- M_min )

Therefore the value quoted for the gradient is m ± Dm

The error in the intercept can be treated in the same way giving

Dc = ½ (c_max- c_min )

Hence this value is recorded as c ± D c

Obviously you need to keep a careful eye on the number of significant figures used and also try to make sure that you don't include any points which are known to be in error.

If a point seems to be too far away from the trend shown by the others either go back and check it or consider leaving it out. If you leave it out of your graph you should still record it in your results table and comment on why you have omitted it from the graph.

The values for the quantities on the x and y axes will have an error associated with them and this can be shown on the graph by the use of error bars. Rather than simply plotting a point lines are added to show the error range.