Diffraction - Multiple Choice Answers

Q1. Monochromatic light of wavelength 590 nm is incident normally on a plane diffraction grating having 4 × 10⁵ lines m⁻¹ .

An interference pattern is produced.

What is the highest order visible in this interference pattern?

A	2
B	3
C	4
D	5

d= ¹/_{(number of lines per metre)} = 1/(4 x 10⁵) = 0.25 x 10^-5

Biggest possible θ would be 90^o - so sin θ would be 1

n therefore would be d/λ = 0.25 x 10^-5/(590 x 10^-9) = 4.23

Only whole numbers are possible for n - so the answer is 4 - choice C

Q2. Light of wavelength λ is incident normally on a diffraction grating of slit separation 4λ.

What is the angle between the second order maximum and third order maximum?

A	14.5°
B	18.6°
C	48.6°
D	71.4°

          

Angles are measured from the line drawn perpendicular to the grating.

We therefore have to find θ₂ for n=2 and θ₃ for n=3, and then find the difference between the two angles.

sin θ = n x λ/d

sin θ₂ = 2 x ^λ/_4λ= 2/4 = 0.50

sin θ₃ = 3 x ^λ/_4λ= 3/4 = 0.75

So, θ₂ = sin^-1 0.50 = 30.0^o

and θ₃ = sin^-1 0.75 = 48.6^o

So the angle is 48.6^o- 30.0^o = 18.6^o - choice B

Q3. Monochromatic light of wavelength 490 nm falls normally on a diffraction grating that has 6 × 10⁵ lines per metre.

Which one of the following is correct?

A	The first order is observed at angle of diffraction of 17°.
B	The second order is observed at angle of diffraction of 34°.
C	The third and higher orders are not produced.
D	A grating with more lines per metre could produce more orders.

 

d = ¹/_{(number of lines per metre)} = ¹/(6 × 10⁵) = 1.67 x 10^-6

sin θ = n x λ/d

sin θ₁ = 1 x (490 x 10^-9)/( 1.67 x 10^-6) = 0.293

sin θ₂ = 2 x (490 x 10^-9)/( 1.67 x 10^-6) = 0.587

θ₁ = 17^o (so A is true)

θ₂ = 36^o (so B is not true)

sin θ₃ = 3 x (490 x 10^-9)/( 1.67 x 10^-6) = 0.880

θ₃= 62^o (so C is not true)

n ∝ d

d = ¹/_{(number of lines per metre)}

so, n ∝¹/_{(number of lines per metre)}

so increasing the number of lines per meter would no produce more orders but less. (so D is not true)

 

Q4. Light of wavelength λ is incident normally on a diffraction grating for which adjacent lines are a distance 3λ apart.

What is the angle between the second order maximum and the straight-through position?

'adjacent lines are a distance 3λ apart' this means that d = 3λ

n = 2

sin θ = n x λ/d

sin θ = 2 x ^λ/_3λ = 0.667

θ = 41.8^o - rounds to 42^o - choice C

Q5.

The diagram above shows the first four diffraction orders each side of the zero order when a beam of monochromatic light is incident normally on a diffraction grating of slit separation d.

All the angles of diffraction are small.

The grating is then exchanged for one with a slit separation ^d/₂

Select the diffraction orders now observed from the patterns, A to D, drawn below. The diagrams are drawn on the same scale as the graphic above.

     

  

  

There is a triangle formed by the diffracted ray, the centre line and the screen.

The sine of the angle of diffraction = the distance from the centre line to the order observed on the screen and the refracted ray.

As the question tells us that the angles are very small we can assume that the length of the path of the diffracted ray is as good as the same for all paths... let us call it L

sin θ = distance between an order and the central line/L

sin θ ∝ 1/d for a given wavelength and order, so halving d would double sin θ

This would increase the spacing between the orders of diffraction observed on the screen.

Choice C is of the same dimensions as the original graphic - so the only possible one is D

Q6. A light source emits light which is a mixture of two wavelength, λ₁ and λ₂.

When the light is incident on a diffraction grating it is found that the fifth order of light of wavelength λ₁ occurs at the same angle as the fourth order for light of wavelength λ₂.

If λ₁ is 480 nm what is λ₂?

A	400 nm
B	480 nm
C	600 nm
D	750 nm

 

sin θ = n x λ/d

sin θ = 5 x λ₁/d = 4 x λ₂/d

λ₂= ⁵/₄ x λ₁ = 1.25 x 480 = 600 nm - Choice C

Q7. A diffraction pattern is formed by passing monochromatic light through a single slit.

If the width of the single slit is reduced, which of the following is true?

	Width of central maximum	Intensity of central maximum
A	unchanged	decreases
B	increases	increases
C	increases	decreases
D	decreases	decreases

 

If you decrease the aperture that the light passes though (the slit width) it is obvious less light will get through - so the intensity at the central maximum will decrease.

With diffraction (from GCSE knowledge!) you know that the narrower the aperture the greater the spread - therefore the width of the central maximum will increase.

Only choice C has both of these effects described.

For a mathematical treatment (no longer on the AQA syllabus) see the page on single slit

W_c = 2D( λ/a)

The central fringe is twice as wide as each of the outer fringes (measured from minimum to minimum intensity)

It is inversely proportional to the size of the aperture 'a'. Therefore decreasing 'a' will increase the width of the central maximum.

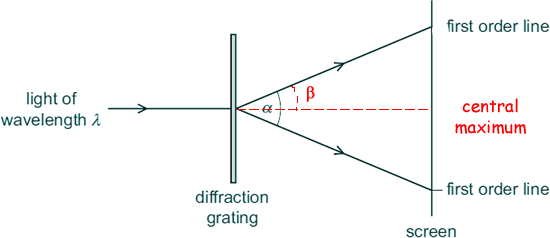
Q8. Light of wavelength λ passes through a diffraction grating with slit spacing d. A series of lines (fringe pattern) is observed on a screen.

What is the angle α between the two first order lines?

d sin θ = n λ - diffraction grating equation

n = 1 (first order line)

2β = α

now, sin β = λ/d

so β = sin^-1( ^λ/_d )

∴α = 2 sin^-1( ^λ/_d )

 

A	sin-1( λ/2d )
B	sin-1( λ/d )
C	2sin-1( λ/2d )
D	2sin-1( λ/d )

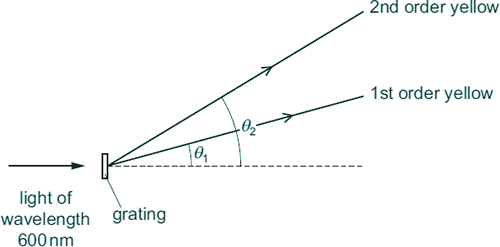
Q9. A diffraction grating experiment is set up using yellow light of wavelength 600nm.

 

The grating has a slit separation of 2.00 μm.

What is the angular separation (θ₂ – θ₁) between the first and second order maxima of the yellow light?

A	17.5°
B	19.4°
C	36.9°
D	54.3°

The diffraction grating equation:

d sin θ = n λ

d sin θ₁ = λ

sin θ₁ = 600 x 10^-9 /2.00 x 10^-6 = 0.30

θ₁ = 17.45°

d sin θ₂ = 2 λ

sin θ₂ = 0.60

θ₂ = 38.87°

θ₂ - θ₁ = 19.42° - Choice B

 

Q10. A wave is diffracted as it passes through an opening in a barrier.

The amount of diffraction that the wave undergoes depends on both the:

A	amplitude and frequency of the incident wave.
B	wavelength and amplitude of the incident wave.
C	wavelength of the incident wave and the size of the opening.
D	amplitude of the incident wave and the size of the opening.

 

Q11. Which of the following provides evidence that light has a wave nature?

A	The emission of light from an energy-level transition in a hydrogen atom.
B	The diffraction of light passing through a narrow opening.
C	The absorption of ultra-violet radiation in the photoelectric effect.
D	The reflection of light from a mirror

Q12. In a diffraction-grating experiment the maxima are produced on a screen.

What causes the separation of the maxima of the diffraction pattern to decrease?

The fringe spacing equation:

w = λD/s

λ and D increasing will make w increase....

Increasing the distance between the source and grating will have no effect other than reduce the light intensity getting through....

Increasing s will reduce w

 

Q13. Two waves with amplitudes a and 3a interfere.

The ratio of (amplitude at interference maximim)/(amplitude at interference minimum) is:

A	2
B	3
C	4
D	infinity

Amplitude at maximum is the sum of amplitude - 4a

Amplitude at the minimum is difference in amplitude - 2a

4a/2a = 2

Q14. White light passes through a single narrow slit and illuminates a screen.

What is observed on the screen?

See here for notes on this.

 

Q15. When light of wavelength 5.0 × 10⁻⁷ m is incident normally on a diffraction grating the fourth-order maximum is observed at an angle of 30°.

What is the number of lines per mm on the diffraction grating?

d sin θ = nλ

 d is the distance between the slits

 θ is the angle of diffraction = 30^o

 λ is the wavelength of the light = 5.0 × 10⁻⁷m

 n is the order of diffraction = 4

d sin 30^o = 4 x 5.0 × 10⁻⁷

d = 4 x 5.0 × 10⁻⁷/sin 30^o

d = 4 x 10^-6 m

number per mm = 10^-3/(4 x 10^-6)

number per mm = 250 (Choice A)

Q16. Intensity maxima are produced on a screen when a parallel beam of monochromatic light is incident on a diffraction grating.

Light of a longer wavelength can be used or the distance from the diffraction grating to the screen can be increased.

Which row gives the change in appearance of the maxima when these changes are made independently?

 

w = λD/s

So, w is proportional to each of them.

Choice B

Q17. Light of wavelength 500 nm is passed through a diffraction grating which has 400 lines per mm.

What is the angular separation between the two second-order maxima?