Q2.

(a)

(i) What is meant by the random nature of radioactive decay?

Which atom decays at what time is pure chance

You cannot predict which atom will decay next. We can only predict how many will decay within certain time time frame by using the mathematics of probability (which requires a large number of events to be reliable).

(ii) Explain what is meant by each of the following:

• isotopes

Isotopes are (different forms) of same element. They have the same proton number, Z, nut a different nucleon number, A [or have same number of protons but different number of neutrons]

• radioactive half-life

The half-life of an isotope is time taken for the number of nuclei (of that particular isotope ) to halve

[OR for the activity (of that isotope ) to halve ]

You must specify that half-life relates to the acivity or count from a particular isotope for the two marks. A sample usually contains several radioactive isotopes - therefore the 'count' from that sample is a combination of all of their decays.

• radioactive decay constant

There are two ways in which you can answer this to get the two marks:

Method 1

dN/dt = –N mathematically expresses that the rate of decay of nuclei of an isotope is proportional to the number of undecayed nuclei;

where is the constant of proportionality that is called the radioactive decay constant.

Method 2

The radioacive decay constant expresses the probability of decay in unit time.

( 6 marks)

(b) The radioactive isotope of iodine ¹³¹I has a half-life of 8.04 days. Calculate

(i) the decay constant of ¹³¹I,

You need to change the time into seconds:

(8.04 x 24 x 60²) = ln 2/

= ln 2/ (8.04 x 24 x 60²) = 1.0 x 10^-6 s^-1

Don't forget the unit!

(ii) the number of atoms of ¹³¹I necessary to produce a sample with an activity of 5.0 × 10⁴ disintegrations s^–1 (Bq),

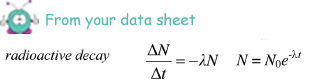
5.0 × 10⁴ = - x N

N = - 5.0 × 10⁴/

N = - 5.0 × 10⁴/(answer to part (i))

N = - 5.0 × 10⁴/1.0 x 10^-6

N = 5.0 x 10¹⁰

(iii) the time taken, in hours, for the activity of the same sample of ¹³¹I to fall from 5.4 × 10⁴ disintegrations s^–1 to 5.0 × 10⁴ disintegrations s^–1

Using the second equation we can replace the N with A by substituting and cancelling the lambdas.

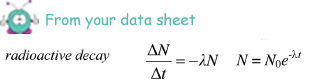
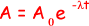
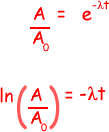
ln (5.0 × 10⁴/ 5.4 × 10⁴) = - t

- 0.077 = -1.0 x 10^-6t

t = 0.077/1.0 x 10^-6 = 7.7 x 10⁴s

7.7 x 10⁴/60² = 21 hours

(6 marks)

(Total 12 marks)