Topic Menu

Cyber-chess a chess instruction site
Gravitational Fields Structured Questions

Q3.

(a)

(i) Explain what is meant by the gravitational field strength at a point in a gravitational field.

The gravitational field strength at a point is the force per unit mass acting on a small test mass placed at that point in the field.

In questions like this they do not want you to simply copy an equation from the data sheet - they want you to explain that equation in words - the minimum you can get away with is quoteing the equation and fully explaining the terms in it!

(ii) State the SI unit of gravitational field strength.

N kg−1

'g' is used to represent two things - 'graviational field strength' and 'acceleration due to gravity'. It has the same numerical value in both meanings. Treated as an acceleration, the unit is m s−2. Treated as a field strength, the unit is N kg−1. You will therefore not get a mark for giving the units of acceleration even though they are equivalent to each other.

(2 marks)

(b) Planet P has mass M and radius R. Planet Q has a radius 3R. The values of the gravitational field strengths at the surfaces of P and Q are the same.

(i) Determine the mass of Q in terms of M.

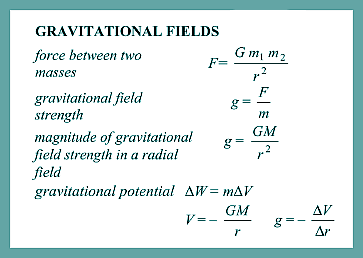
gP = gQ and G is a constant

so Mp/rp2 = Mq/rq2

Mq = Mprq2/rp2

Mp = M

rq = 3R

rp = R

Mq = M(3R)2/R2

Mq = 9M

 

(ii) The graph shows how the gravitational field strength above the surface of planet P varies with distance from its centre.

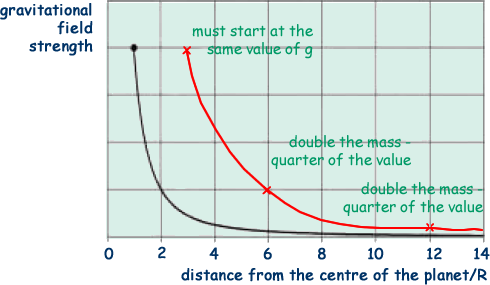
An a line to the graph to show the variation of the gravitational field strength above the surface of Q over the range shown.

You have worked out that planet Q has 9 times the mass of planet P.

At any position in space, gQ will be 9 times greater than gP becasue g ∝ M.

Your curve must start at 3R, as this is the surface radius of Q,

Graph line

  • starts at 3R, with same initial value of g as existing curve
  • is a curve of decreasing negative gradient
  • shows the correct inverse square relationship,

(6 marks)

(Total 8 marks)