Topic Menu

Cyber-chess a chess instruction site

Circular Motion

Q1.

(a) Derive an expression to show that for satellites in a circular orbit T2 ∝ r3 where T is the period of orbit and r is the radius of the orbit.

Fcentripetal = mv2/r

v = circumference of orbit/period of orbit

v = 2πr/T

Fcentripetal = m (2πr/T) 2/r

Fcentripetal = mr (2π/T) 2

The centripetal force is provided by the gravitational force

Fgravitational= GmM/r2

∴  GmM/r2 = mr (2π/T) 2

GM/r3 = (2π/T) 2

T2 = r3 x 4π2/(GM)

m cancelled - π, G and M are constant

so T2 ∝ r3 Q.E.D.

[2 marks]

(b) Pluto is a dwarf planet.

The mean orbital radius of Pluto around the Sun is 5.91 × 109 km compared to a mean orbital radius of 1.50 × 108 km for the Earth.

Calculate in years the orbital period of Pluto.

T2 ∝ r3

TP2 /rP3= TE2 /rE3

TP2 = TE2 rP3/rE3

TP2 = 12 x (5.91 × 109)3/(1.50 × 108 )3

TP2 = 61160

TP = 247 years

[2 marks]

(c) A small mass released from rest just above the surface of Pluto has an acceleration of 0.617 m s−2.

Assume Pluto has no atmosphere that could provide any resistance to motion.

Calculate the mass of Pluto.

Give your answer to an appropriate number of significant figures.

radius of Pluto = 1.19 × 106 m

Fgravitational= GmM/r2 = ma

a = GM/r2

M = ar2/G

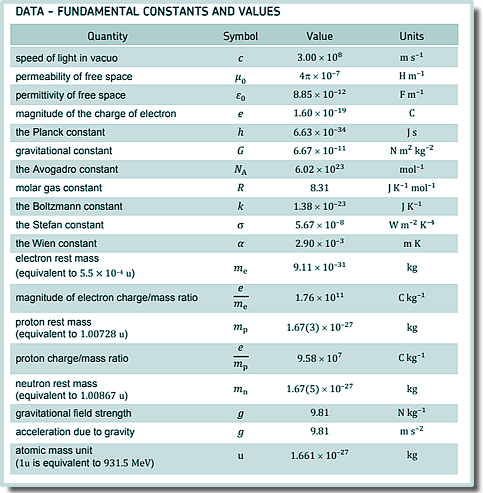
M = 0.617 x (1.19 × 106)2 /6.67 x 10-11

M = 1.31 (three sig figs ) x 1022 kg

[3 marks]

(d) The graph shows the variation in gravitational potential with distance from the centre of Pluto for points at and above its surface.

A meteorite hits Pluto and ejects a lump of ice from the surface that travels vertically at an initial speed of 1400 m s−1 .

Determine whether this lump of ice can escape from Pluto.

Let the mass of the ice be mice

Initial KE = ½ micev2

Initial KE = ½ mice x 14002

Initial KE = ½ mice x 14002

Initial KE = mice x 9.8 x 105 J

See the graph:

Energy needed to escape = mice x 7.4 x 105 J

Therefore there is sufficient energy to escape.

OR

You could work out the escape velocity

For object on surface to escape kinetic energy must equal the gravitational potential energy

7.4 x 105 x mice= ½ micev2

escape speed = √(7.4 x 105 x 2)

escape speed = 1220 ms-1

So sufficient (initial) speed to escape.

[3 marks]

(10 marks total)