Topic Menu

Cyber-chess a chess instruction site

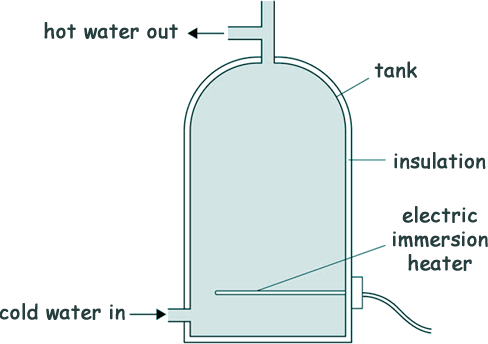
Heat Questions - GCSE Level

Q10. The diagram shows a hot water tank made of copper.

(a) Copper has a higher thermal conductivity than most metals.

What does that tell us about the rate of energy transfer through copper compared with the rate of energy transfer through most metals?

A
 it is higher
B
 it is lower
C
 it is the same

[1 mark]

(b) When the water is hot, the immersion heater switches off. The tank is insulated.

(i) How does the rate of energy transfer from the water in an insulated tank compare to a tank with no insulation?

It is lower - the insulation makes it more difficult for heat to travel to the surroundings.

(ii) How does having a water tank insulated affect the time taken to heat a full tank of water?

It is quicker to heat an insulated water tank as the heat energy is held within the tank more efficeintly than in a non-insulated one.

(iii) How does having a water tank insulated affect the time that the water stays hot within the taken after being heated?

An insulated water tank stays hot longer as the heat energy is held within the tank more efficeintly than in a non-insulated one.

(3 marks)

(c)

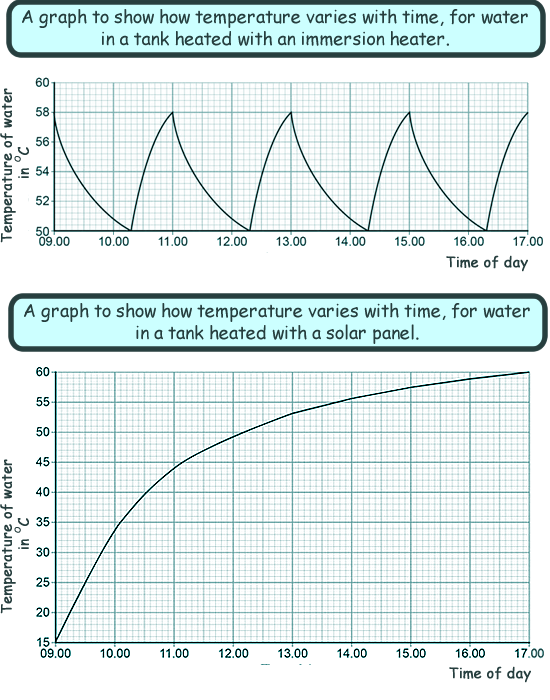
Using only the information from the graphs avove, give one advantage and one disadvantage of heating the water using solar panels rather than an immersion heater.

The advantage is that the water heated continuously (by the Sun) throughout the day.

The disadvantage is that you have less control over water temperature. The temperature of water is lower (for most of the time than water heated by immersion heater) because it takes longer to heat the water , and therefore the water may not be hot enough for you to use in the morning.

You only get only one mark for advantage and one for disadvantage.

Note that you only get marks for what you can glean from the graphs - so saying how solar energy is 'free after instalation' etc will not get any marks!

[2 marks]

(d) During one morning, a total of 4,070,000 J of energy is transferred from the electric immersion heater. 4,030,000 J of energy are transferred to the water.

Calculate the proportion of the total energy transferred to the water.

4030000/4070000 = 0.99

99% of the energy is transferred to the water.

 

[2 marks]

(e) The power output of the immersion heater is 5000 W.

(i) Write down the equation that links energy transferred, power and time.

energy = power x time

or

P = E/t

[1 mark]

(ii) Calculate the time taken for the immersion heater to transfer 4 070 000 J of energy.

time = energy/power

t = E/P

t = 4070000/5000 = 814 seconds

(t = 13 minutes 34 seconds)

[3 marks]

(12 marks total)