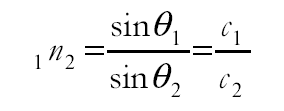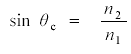Topic Menu

Cyber-chess a chess instruction site
Snell's Law

This gives a mathematical relationship to the observations made at GCSE.

I talk in terms of OPTICAL media on this page - but there is nothing to stop the examiners talking about sound - remember is is the SPEED CHANGE that makes refraction occur - look carefully at the basic refraction page and follow the link to geophysics and refraction of sound. You must UNDERSTAND what is happening!

If you carry out an experiment and measure the angle of incidence and the angle of refraction you will find that if you plot the sine of the angle of incidence against the sine of the angle of refraction you get a straight line that goes through the origin. This indicates that the sine of the angle of incidence is directly proportional to the sine of the angle of refraction and that the ratio of the two will produce a constant value that we call the refractive index. The ratio of the sines of the angles is equal to the ration of the speeds of the waves through the media.

Snell's Law states that the ratio of the angle of incidence to the angle of refraction of a wave as it travels through a boundary between two media is a constant termed the refractive index. The value of this constant is equal to the ratio of speeds before and after it crosses the boundary.

1n2 is the inverse of 2n1...... to swap them round you simply find the reciprocal (see interactive spreadsheet)

Remember - if it slows down the the angle of refraction will be smaller than the angle of incidence and therefore the ratio will equal a value greater than 1.... and vice versa. Always sort out what is happening to the speed of the wave as it crosses into the new medium.

Critical Angle

If the angle of refraction is 90o then the angle of incidence is called the critical angle.

The sine of 90o is 1 so the equation becomes:

sine of the critical angle = the refractive index going from an optically dense to an optically rare medium (a less than 1 value!!!)

BUT the refractive index is usually given the other way round! ....

in this equation 'n' is quoted for rays going from the rare optical medium to the denser one (slowing down!)

If a ray is hitting a boundary between two media (like in a fibre optic thread when it meets the cladding) then you use this equation (on your data sheet)

  • Here is a link to the interactive spreadsheet on this topic.
  • Here is a link to a page of questions for you to try - the answers are given to you.... only see me about the ones you CAN'T do!

 

LOJ April 2003