Solutions: Radioactivity Questions
Q5. Potassium-42 decays with a half-life of 12 hours. When potassium-42 decays, it emits β– particles and gamma rays. One freshly prepared source has an activity of 3.0 × 107 Bq.
(a) To determine the radiation dose absorbed by the scientist working with the source, the number of gamma rays photons incident on each cm2 of the body has to be known.
One in every five of the decaying nuclei produces a gamma ray photon.
A scientist is initially working 1.50 m from the fresh source with no shielding. Show that at this time approximately 21 gamma photons per second are incident on each cm2 of the scientist's body.
Surface area of a sphere is the area that intercepts them. So, at 1.5 m from the source:
3.0 × 107x 0.2
/(4 π
r2) gamma rays fall on each m2
= 2.1 x 105
Therefore on each cm2:
2.1 x 105/104 = 21 Q.E.D.
(3 marks)
(b) The scientist returns 6 hours later and works at the same distance from the source.
(i) Calculate the new number of gamma ray photons incident per second on each cm2 of the scientist's body.
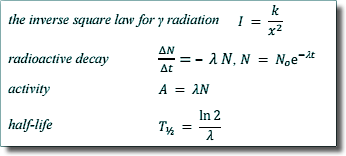
Thalf = ln 2/λ
λ = ln 2/Thalf
λ = ln 2/12 = 0.0578 hour-1
OR (changing to seconds) λ = ln 2/(12 x 602) = 1.60 x 10-5 s-1
N = N0e-λt
∴ A = A0e-λt
So, after 6 hours:
A = 3.0 × 107e-(0.0578 x 6) or (changing to seconds) A = 3.0 × 107e-(1.60 x 10-5 x 6 x 60 x 60)
A = 3.0 × 107e-0.346
A = 3.0 × 107x 0.708 = 2.12 × 107
Therefore the multiplication factor for activity and number of gamma rays hitting the scientist is 0.708
Therefore 21 x 0.708 = 15 gamma rays per second hit each cm2 of the scientist per second after six hours.
OR a really quick way....
6 hours is 0.5 half-lives
The source activity decreases to 2–0.5 of initial activity in this time
So, the new no. of gamma photons per second per cm2 = 21.2 × 2–0.5= 15
(ii) Explain why it is not necessary to consider the beta particle emissions when determining the radiation dose the scientist receives.
Any two of the following points:
beta particle range in air is less than 1.5m
beta particles are absorbed by air
beta particles lose energy in air much more rapidly than gamma photons
beta particles ionise air much more than gamma photons
(5 marks)
(Total 8 marks)
About this site
