Solutions: Radioactivity Questions
Q13.
(a) In a radioactivity experiment, background radiation is taken into account when taking corrected count rate readings in a laboratory. One source of background radiation is the rocks on which the laboratory is built. Give two other sources of background radiation.
Any 2 from: 

- the sun,
- cosmic rays,
- radon (in atmosphere),
- nuclear fallout (from previous weapon testing) ,
- any radioactive leak (may be given by name of incident)
- nuclear waste,
- carbon-14 in the atmosphere
(1 mark)
(b) A γ ray detector with a cross-sectional area of 1.5 × 10–3m2 when facing the source is placed 0.18 m from the source. A corrected count rate of 0.62 counts s–1 is recorded.
(i) Assume the source emits γ rays uniformly in all directions. Show that the ratio number of γ photons incident on detector to the number of γ photons produced by source is about 4 × 10–3.
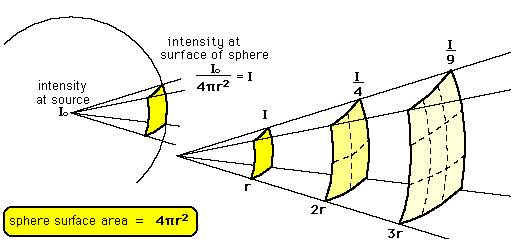
Let number of gamma rays emitted be N
N gamma rays would spread out over a sphere of surface area 4πr2= 4π x 0.182m2
But the number detected would be only part of that - it would be 1.5 × 10–3m2
(1.5 × 10–3)/(4π x 0.182) = 0.00368 
This is approximately 4 × 10–3 QED
(2 marks)
(ii) The γ ray detector detects 1 in 400 of the γ photons incident on the facing surface of the detector. Calculate the activity of the source. Give an appropriate unit.
0.62 s–1 are detected.
If every one could be counted that would be 0.62 x 400 = 248 s–1
From part (b) we calculated that only 0.00368 of the output was detected because the counter only intercepted a small section of the emitted rays.
Therefore the initial emision was 0.62 x 400/0.00368 = 67 kBq
kBq
(3 marks)
(c) Calculate the corrected count rate when the detector is moved 0.10 m further away from the source.
I = k/x2
Therefore I1x12 = I2x22
I2 = I1x12/x22
I2 = 0.62 x 0.182/0.282
I2 = 0.26 counts s–1
(3 marks)
(Total 9 marks)


