Topic Menu

Cyber-chess a chess instruction site

Gravitational Fields Questions

Q4.

(a) By considering the force equation for a satellite of mass m in an orbit of radius r around a planet of mass M, show that the orbital time period T of the satellite does not depend upon m.

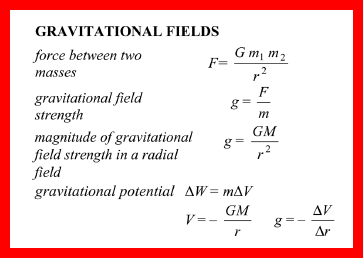
The gravitational force forms the centripetal force that holds the satellite in orbit.

GMm/r2 = mv2/r

GM/r= v2

'm' cancels out

Now v = 2πr/T

so, GM/r = 4π2r2/T2

T2= 4π2r3/GM

∴ T = 2π(r3/GM)0.5

m does not figure in the expression so T is independednt of m QED

(3 marks)

(b) One of the moons of Jupiter, Ganymede, is the largest satellite in the solar system.

Its orbital period is equal to 7.15 Earth days and the radius of its orbit is 1.07 × 106 km. Calculate

(i) the angular speed of Ganymede in its orbit,

ω = 2π/T

ω = 2π/(7.15 x 24 x 602)

ω = 1.02 x 10-5 rad s-1

(ii) the centripetal acceleration of Ganymede in its orbit,

r = 1.07 × 106 km = 1.07 × 109 m.

a = ω 2 r = (1.02 × 10-5)2 × 1.07 × 109

a = 0.111 m s-2

(iii) the mass of Jupiter.

When doing a question with (i), (ii), (iii) etc parts, you are usually expected to build on the previous parts.

centripetal acceleration = g = GM/r2

M = gr2/G

M = 0.111 x (1.07 × 109)2/ 6.67 × 10-11

M = 1.91 x 1027 kg

But you could do it another way -

T2= 4π2r3/GM (from part (a))

M = 4π2r3/GT2

M = 4π2(1.07 × 109)3/(6.67 × 10-11(7.15 x 24 x 602)2)

M = 1.90 x 1027 kg

(5 marks)

(Total 8 marks)