Gravitational Fields Questions
Q1.
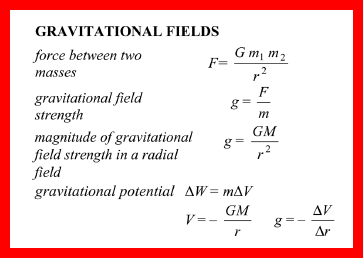
(a) State Newton's law of gravitation.
The force of attraction 
between two point masses (or particles)is proportional to product of their massesand inversely proportional to the square of distance between their centres.
(2 marks)
(b) In 1798 Cavendish investigated Newton's law by measuring the gravitational force between two unequal uniform lead spheres. The radius of the larger sphere was 100mm and that of the smaller sphere was 25mm.
(i) The mass of the smaller sphere was 0.74 kg. Show that the mass of the larger sphere was about 47 kg.
density of lead = 11.3 × 103 kg m–3
density 
= m/V
V of sphere = 4/3
r
3
mass of larger sphere ML = 4/3r
3
= 4/3 × (0.100)3 × 11.3 × 103
ML = 47(.3) kg QED
OR
use of M is proportional to r3
(2 marks)
(ii) Calculate the gravitational force between the spheres when their surfaces were in contact.
r = sum of the radii = 25 + 100 = 125 mm
F = G MLMS/r2
= 6.67 x 10-11 x 47.3 x 0.74 / (125 x 10-3)2
= 1.5 × 10−7 N
(2 marks)
(c) Modifications, such as increasing the size of each sphere to produce a greater force between them, were considered in order to improve the accuracy of Cavendish's experiment.
Describe and explain the effect on the calculations in part (b) of doubling the radius of both spheres.
For the spheres, mass is proportional to the volume (or proportional to the radius cubed therefore doubling the radius of each sphere would make its mass eight times as much.This would make the force of atttraction between them 8 x 8 = 64 times as great.
However the radii being increased would double the distance between the centres of the masses. This having an inverse square relationship would reduce the force of attraction by a factr of four.
The overall effect would therefore be 64/4 = 16 times as great.
(4 marks)
(Total 10 marks)
About this site
