Quantum Phenomena - discrete energy levels for electrons
Q2. Some energy levels of an atom of a gas are shown in diagram 1.

Diagram 1
When a current is passed through the gas at low pressure, a line spectrum is produced. Two of these lines, which correspond to transitions from levels B and C respectively to the ground state, are shown in diagram 2.
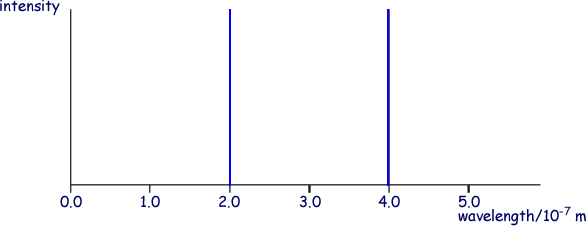
Diagram 2
(a) Describe what happens to an electron in an atom in the ground state in order for the atom to emit light of wavelength 4.0 × 10–7 m.
The ground state electron needs to be excited/promoted to a higher energy level/orbit.  This is achieved by the interaction of a high energy electron with the electron in the ground state (OR irradiation by elecromagnetic energy)
This is achieved by the interaction of a high energy electron with the electron in the ground state (OR irradiation by elecromagnetic energy)  When the excited electron relaxes/is de-excited/falls back to a lower energy state a photon of electromagnetic radiation is emitted.
When the excited electron relaxes/is de-excited/falls back to a lower energy state a photon of electromagnetic radiation is emitted.  The photon has an energy that corresponds to the energy jump between the levels. The photon's wavelength is related to that energy by the equation E = hf = hc/λ
The photon has an energy that corresponds to the energy jump between the levels. The photon's wavelength is related to that energy by the equation E = hf = hc/λ
(3 MAX marks)
(b) Determine the energy, in J, of
(i) the photons responsible for each of the two lines shown in diagram 2.
E = hf = hc/λ
400 nm line
E = 6.63 x 10-34 x 3.0 x 108/4.0 × 10–7 m
E = 4.95 x 10-19 J
E = 5.0 x 10-19 J
200 nm line
E = 6.63 x 10-34 x 3.0 x 108/2.0 × 10–7 m
E = 9.9 x 10-19 J
(ii) the energy of each of levels B and C in Figure 1.
 Transition from A to B is 5.0 x 10-19 J = 0.5 x 10-18 J
Transition from A to B is 5.0 x 10-19 J = 0.5 x 10-18 J
Therefore the energy of level B =- (2.0 + 0.5) x 10-18 = – 1.5 × 10–18 J 
 Transition from A to C is 10.0 x 10-19 J = 1.0 x 10-18 J
Transition from A to C is 10.0 x 10-19 J = 1.0 x 10-18 J
Therefore the energy of level C =- (2.0 + 1.0) x 10-18 = – 1.0 × 10–18 J 
(5 marks)
(Total 8 marks)








