Quantum Phenomena - discrete energy levels for electrons
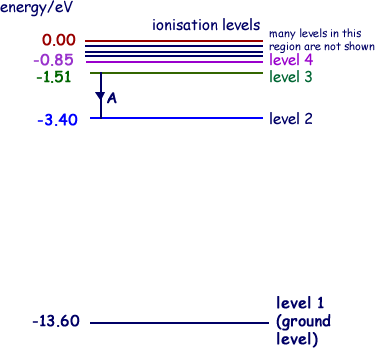
Q1. The diagram below shows the energy level diagram of a hydrogen atom.
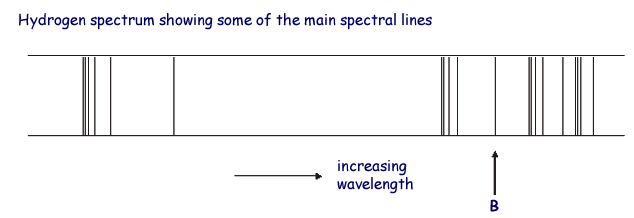
The associated spectrum to the diagram above is
shown below.
The transition labelled A in the top diagram gives the spectral line labelled B in the spectrum diagram.
(a)
(i) Show that the frequency of spectral line B is about 4.6 × 1014 Hz.
ΔE = 3.40-1.51 = 1.89 eV
ΔE = 1.89 × 1.60 × 10–19J 
ΔE = 3.02 × 10–19 J
For the emitted photon E = hf so
f = E/h = 3.02 × 10–19 /6.63 x 10-34 = 4.56 × 1014 Hz
(ii) Calculate the wavelength represented by line B
c= fλ so
λ =c/f
λ = 3.0 x 10-8/4.56 × 1014
λ = 6.58 × 10–7 m
(3 marks)
(b) The hydrogen atom is excited and its electron moves to level 4.
(i) How many different wavelengths of electromagnetic radiation may be emitted as the atom returns to its ground state?
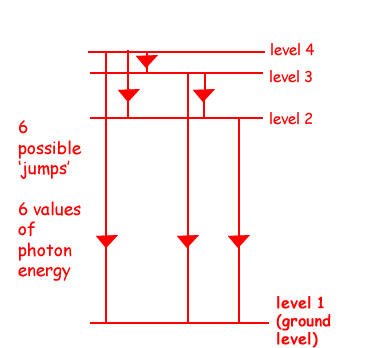
6 different wavelengths of electromagnetic radiation may be emitted
(ii) Calculate the energy, in eV, of the longest wavelength of electromagnetic radiation emitted during this process.
–0.85 - (-1.51) = -0.85 + 1.51
= 0.66 eV
(2 marks)
(c) In a fluorescent tube, explain how the mercury vapour and the coating of its inner surface contribute to the production of visible light.
Marks were awarded for a maximum of 3 of the follwoing points:
- mercury vapour at low pressure is conducting
- atoms of mercury are excited by electron impact
- producing (mainly) ultra violet radiation
- which is absorbed/ excites the coating
- which, upon relaxing, produces visible light
- electrons cascade down energy levels
(3 marks)
(Total 8 marks)
About this site
