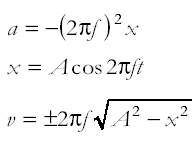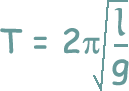The constant of proportionality is w²

A strange constant you may think! .... but, in order to understand where it comes from we have to do a bit of mathematical analysis.

w is the angular velocity of the circular motion that corresponds to the SHM. It is equal to 2pf where f is the frequency of the sinusoidal waveform that is associated with SHM. (See circular motion).

Now, at what point should we begin timing? Does it matter? Well, no, not really, the motion is still SHM! But the mathematical desription of the motion will alter depending on where we begin the cycle!

If we time from the centre of the oscillation (where x=zero at time t=0s) we get a sine curve to describe the displacement as time varies, if we time from a point where x=A (maximum displacement) we get a cosine curve, but either way, after a bit of mathematical analysis, we get rid of the original trig function for displacement and get 'a' in terms of 'x' and a constant!

Let me show you how:

Mathematical treatment 1 (t=0 when x=0)

In a graph of displacement against time for a particle exhibiting SHM the amplitude of the graph would be A and the equation would be:

x = A sin wt

The differential (gradient with respect to time) of this equation would give the velocity 'v'.

v = A w cos wt

The differential (gradient with respect to time) of this equation would give the acceleration 'a'.

a = -A w² sin wt

BUT

Asinwt = x

so

a = -Aw²sinwt = -w²x

Mathematical treatment 2(t=0 when x=A) - this is the one AQA give you on the data sheet!

In a graph of displacement against time for a particle exhibiting SHM the amplitude of the graph would be A and the equation would be:

x = Acoswt

The differential (gradient with respect to time) of this equation would give the velocity 'v'.

v = -Awsinwt

The differential (gradient with respect to time) of this equation would give the acceleration 'a'.

a = -Aw²coswt

BUT

A cos wt = x

so

a = -Aw²coswt = -w²x

BOTH treatments have the same result! Care when tackling questions that you choose the correct trig function (the one in the syllabus is the cosine one... but in Lowe and Rounce you many need to use the sine one occasionally) to describe the situation given in the question - look to what is happening when t=0s!!

Lots of natural systems exhibit SHM - bouncing, swinging, vibrating things are all usually moving in SHM: from atomic vibrations to suspension bridges!

Note that the equation has acceleration 'a' proportional to the negative value of the displacement' x' (i.e. proportional to the distance (magnitude of the displacement!) but opposite in direction - acceleration increases as it gets closer to the point we are measuring from. The constant of proportionality is linked to the frequency 'f' of the oscillation. Therefore the general equation for SHM could be written:

a = - (2pf)²x

Now

v = - Awsinwt

So

v² = A²w²sin²wt

But

sin²wt + cos²wt = 1

so

v² = A²w² (1-cos²wt)

v² = A²w² - A²w²cos²wt

However we know that:

Acoswt = x

Squaring this we get:

A²cos²wt = x²

Therefore, by substituting it into our equation we get:

v² = A²w² - w²x²

And taking the square root of this we get:

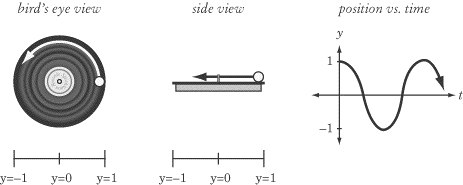
v = +w(A² - x²)^0.5

or

You are GIVEN this on the data sheet - you do not have to worry about deriving it - this just shows where it comes from (same thing can be done with the sine expression... try it!)

This equation gives the velocity at any displacement x.

If it is at the extremities x=A therefore the velocity is zero.

At the centre of the oscillation x=0 so v= wA=2pfA (maximum velocity) the +/- indicates the direction of travel.

You should be able to sketch the graphs of displacement, velocity and acceleration against time for a system operating SHM.

If you start at the point x=0 at time t=0 then the displacement graph will be a sine curve (amplitude A), the velocity a cos curve (amplitude wA) and the acceleration a negative sine curve (amplitude w²A) - where w is 2pf.

If you start at a distance A from the point x=0 at time t=0 then the displacement graph will be a cosine curve (amplitude A), the velocity a negative sine curve (amplitude wA) and the acceleration a negative cosine curve (amplitude w²A) - where w is 2pf

Exchange of potential and kinetic energy in oscillatory motion

The energy of the system is taken to be constant - made up of kinetic (max at x, where velocity is a max and zero at A because velocity at A is zero) plus potential which has the opposite characteristics of the kinetic. See this page.

The link between circular motion and SHM

If you observe a body rotating uniformly in a circle from a side perspective (in one plane only) it exhibits SHM - see Java applet.