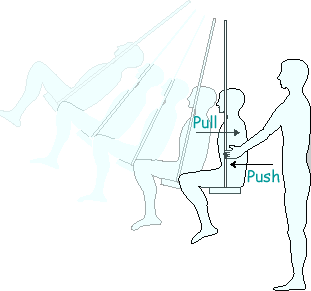When a body at rest is displaced from its equilibrium position by you doing work on it, it gains potential energy.

For example, to start a child swinging on a swing, you either pull the child back wards or push the child forwards. You have to 'do work' to give the child potential energy so the swing will start to 'swing' when released.

When it is released, gravity acts on it and the gravitational potential energy changes into kinetic energy.

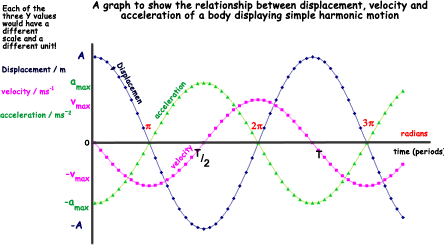
If m is the mass of system executing SHM then the kinetic energy of system at any instant of time is E_K= ¹/₂mv²

E_K = constant x v²

That means that if we plot the kinetic energy of the system against time we will get a graph that has the shape of the velocity expression for SHM squared.

We know that the graph for velocity is of a sinusoidal form. If we take x as being at maximum displacement at time t then the graph for velocity is a -sine curve

When we square that we get a sine squared waveform - which looks a lot like the sine curve!

The big difference is that the squared form has no negative portion and this results in the frequency of the curve doubling. Let's look at the velocity trace.

.

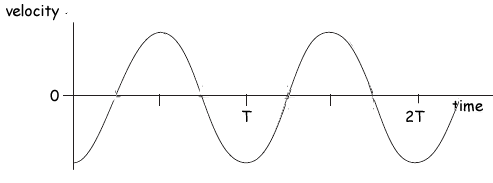Squaring it we get the kinetic energy trace...

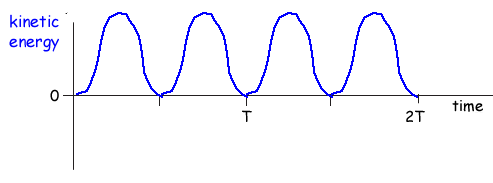
The potential energy (gravitational in the case of a pendulum and stretch energy in the case of a spring) interchanges with the KE... as one increases the other decreases - the sum of both being a constant value (assuming no energy transfer from the system).

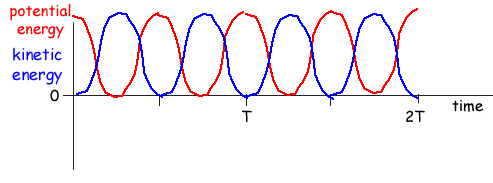
This means that the potential energy trace looks like this:

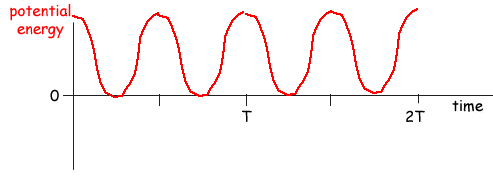
In an examination you will sometimes be asked how the energy changes with time... and sometimes how the energy varies with displacement from the equilibrium position.

If that is the case you will only have a displacement axis with the centre point as the equilibrium position. At this point KE will be a max and PE will be zero... at the extremes the opposite will be true.

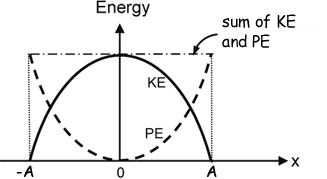
The trace in this case is a curve (the shape of the path of the swing of a pendulum). Make sure you know which you are being asked about - especialy in multiple choice questions!