Go to Main SiteMobile Site IndexMobile Site Index
 
Standing or Stationary Waves on a String

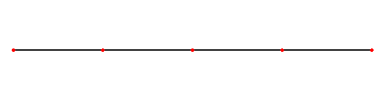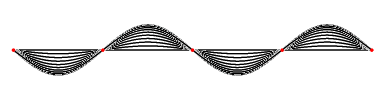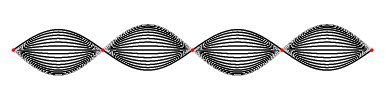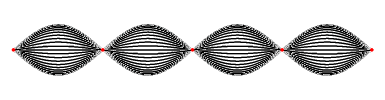
For vibrations in a string (e.g. the plucking of a guitar string) there are two fixed nodes at each end.

Nodes (red dots in the diagram) are points of no vibration and antinodes are points of maximum vibration. Look at the following animation to see how the standing wave envelope is formed...

Now let us consider the length 'L' and its relationship to the wavelength and frequency:

In fundamental frequency (first harmonic) mode we can see that we have half a waveform

L = 1 /2

now as c = f

L = c/(2f1)

and f1= c/(2L)

so the fundamental frequency is half of the ratio of the speed to the length

The speed of the waveform 'c' depends upon the string tension 'T' and the mass per unit length 'μ' of the material the string is made of - see here for derivation - not required at A level.

we can therefore amend our equation to:

(the one in the AQA syllabus)

In a similar way we can examine the harmonic or overtone modes:

for the second harmonic L =

so f2= c/L = 2 f1 (double the frequency of the fundamental - therefore one octave higher)

for the third harmonic L = 3/2

so f3=3c/2L = 3 f1 (triple the frequency of the fundamental)

for the fourth harmonic L = 2

so f4= 2c/L = 4 f1 (double the frequency of second harmonic - therefore one octave higher than that and two octaves higher than the fundamental)

All of the particles between two nodes vibrate in phase with each other but the amplitude of vibration varies from zero at the node to maximum at the antinode back down to zero at the second node.

There are therefore two particles that are in phase and have the same frequency in each 'lobe' of the wave... except for at the antinode where there is just one maximum.

Neighbouring 'lobes' are in antiphase with each other.

Faraday waves (not on the A level syllabus) , also known as Faraday ripples, are nonlinear standing waves that appear on liquids enclosed by a vibrating receptacle. They are named after Michael Faraday, who first described them in an appendix to an article in the Philosophical Transactions of the Royal Society of London in 1831.

If a layer of liquid is placed on top of a vertically oscillating platform, a pattern of standing waves appears which oscillates at half the driving frequency. These waves can take the form of stripes, close-packed hexagons, or even squares or quasiperiodic patterns. Faraday waves are commonly observed as fine stripes on the surface of wine in a wineglass that is ringing like a bell.

Go to Main Site
Mobile Site Index