 |
||||
    |
||||
Cobalt
- 61
|
||||
|
The half-life of cobalt 61 is 100 minutes. How long does it take for the activity of the sample to fall to 80% of its initial value?
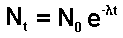
Nt = 0.8N0 t = ? half life = 100 minutes l = ln2/(half life) = 0.693/100 minutes-1 = 0.00693 minutes-1 0.8 = e-lt Take logs ln 0.8 = -lt Substitute for l ln 0.8 = - 0.00693 x t Look up the log -0.223 = - 0.00693 x t t = 32 minutes |
||||
 |
 |
|||
