The Young Modulus is named after Thomas Young (1773 to 1829) who was a British polymath, contributing to our understanding of optics, physiology, and Egyptology, among other fields

The Young Modulus is also known as Young's Modulus or the elastic modulus or tensile modulus.

It is a measure of the stiffness of a material that is independent of the particular sample of a substance. That means a generic value can be given for a material without its dimensions being known (like the values given for resistivity).

It is the ratio of tensile stress (force/cross sectional area) to tensile strain (extension/ original length) - don't forget to put the word 'tensile' into any definition you give in an exam - it will lose you marks!

N.B. AQA no longer expect you to know the symbols for these values.

The unit will therefore be the same as the unit of pressure (Pa or N/m²)

Strains can be quoted in several ways: as a %, or decimal. E.g. a 5% strain = 0.05.

It can be experimentally determined from the slope of a stress-strain curve created during tensile tests conducted on a sample of the material.

• The value of the Young's Modulus is quoted for various materials but the value is only approximate. This is because Young's Modulus can vary considerably depending on the exact composition of the material. For example, the value for most metals can vary by 5% or more, depending on the precise composition of the alloy and any heat treatment applied during manufacture.
• If a big force only produces a small extension then the material is 'stiff' and E is a big value. If a force produces a big extension then the material is not very stiff - it is easier to stretch and the value of E will be smaller.

Linear vs non-linear

For many materials, Young's modulus is a constant over a range of strains. Such materials are called linear, and are said to obey Hooke's law. Examples of linear materials include steel, carbon fiber, and glass. Rubber and soil (except at very low strains) are non-linear materials.

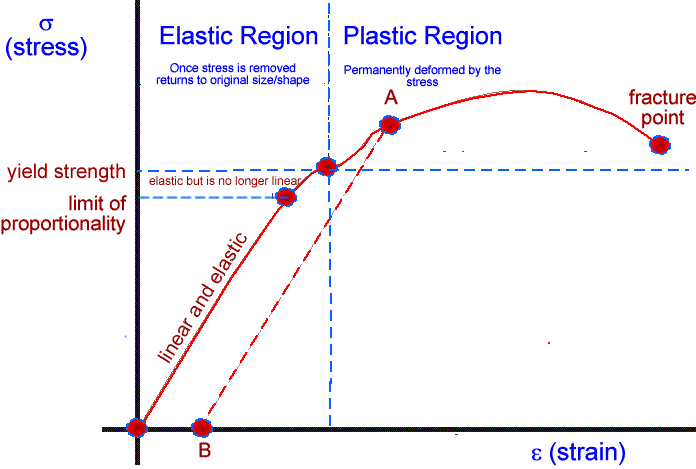
This stretching behavior is summarized in a stress-strain graph such as the one above. As the stress is increased initially Hooke's Law is obeyed - the stress-strain relationship for the wire is linear and elastic.

Just before the plastic region is reached we get the limit of proportionality - beyond this for a small section we see non-linear behaviour but the stretching is still elastic.

After the yield strength, the material enters the plastic deformation region, which means that the stretch of the wire is permanent. (For example, if the wire is stressed to point A on the graph and the stress is slowly decreased, the stress-strain curve follows the dotted line instead of the original curve to point B and there is a permanent extention when all stress is removed.) At the facture point the wire reaches its snaps.

Differences in the shape and limits of the stress-strain diagram determines whether a material is considered ductile or brittle, elastic or plastic.

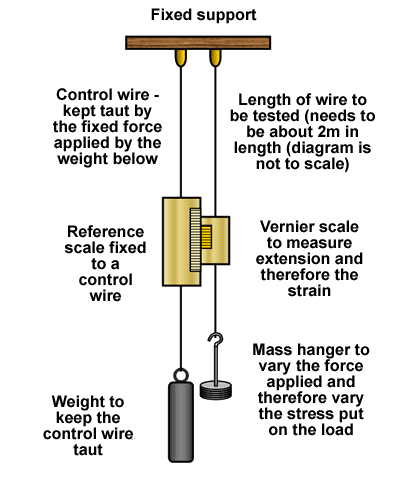
An experiment to measure the Young's Modulus

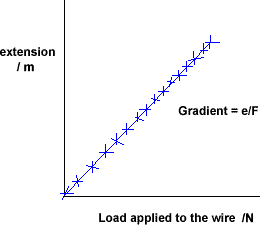
To minimise errors the control wire is the same length, diameter and material as the test wire. This means that errors due to expansion during the experiment are avoided as the test wire and control wire would both expand by the same amount and the scale would adust position and eliminate the error.

The wire must have no kinks in it otherwise there will be big extensions due to the wire straightening out rather than just stretching.

Care must be taken that the limit of proportionality is not exceeded. This can be checked by removing the load after each addition of the weight. If the limit has not been exceeded the wire should return to the length it was before the weight was added.

The wire is as long as possible (usually about 2m long) and it is as thin as possible so that as big an extension as possible can be recorded. (A typical extension for a 5N loading will be 1mm).

Possible Risks

Heavy weights can cause problems if dropped on someone's toe. Care must be taken not to stand so that the masses are over your fooot!

The wire is very thin and taut. It is possible to cut yourslef on it if you walk into it. The area you are working in should be 'fenced off' so that someone doesn't husrt themsleves.

The wire might snap. If it does it might whip into your face and eyes. Goggles should be worn and the area around the wire should be clear so that if you have to move out of the way you can do so quickly.

Procedure

The test wire is loaded with the weight hanger so that it is taut before readings are taken. The vernier scale is read and the result recorded as addition of 0N. Weights  - usually starting at 0N and increasing in 5N increments to 100N - are then added and a reading of the vernier scale is taken at each addition.

The experiment should be repeated twice and any anomalous results repeated and checked.

A graph of extension (Y-axis) against load (X-axis) is plotted. It should be a straight line through the origin.

The gradient of that graph will be e/F. Using that value we can find the value of Young's Modulus for the wire.