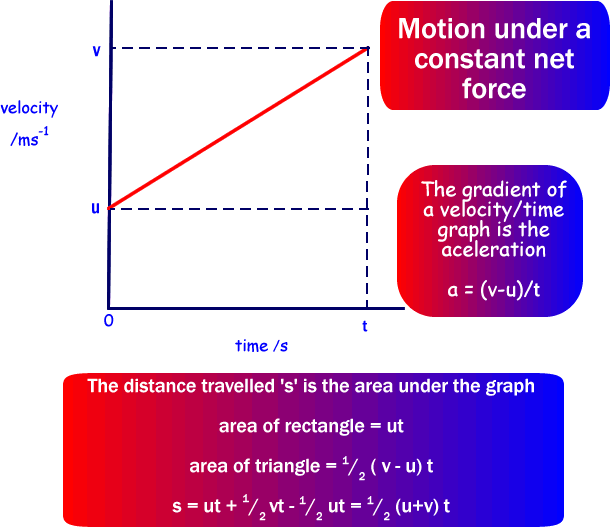
Consider the graphic above. If we plot a graph of an object's motion under the influence of a constant force, we get a graph like the one above.

The initial velocity is u

The final velocity is v

The time of travel is 't'

and the distance travelled in that time is 's'

We get two relationships directly from that graph:

From the gradient we get:

equation 1

v = u + at

From the area under the graph we get

equation 2

s = ¹/₂ (u + v)t

If we replace the v in the second equation with the expression from the first. we get

s = ¹/₂ (u + (u + at))t = ¹/₂ (2u + at))t = (u + ¹/₂at)t

equation 3

s = ut + ¹/₂at²

If we square the first equation we get

v² = (u + at)²= u² + a²t²+ 2uat = u² + 2a (¹/₂at² + ut)

and substitution of (¹/₂at² + ut) as 's' from equation 3 gives us:

equation 4

v² = u² + 2as

The thing to remember about these equations is they can only be applied to a situation in which a constant net force is acting.There has to be constant acceleration for them to apply.

Most examination questions wherein you have to use these equations will concern 'objects falling under gravity' - air resistance has to be negligible for them to be applicable... and you may be expected to state that air resistance is being ignored...