There are lots of different types of graph:

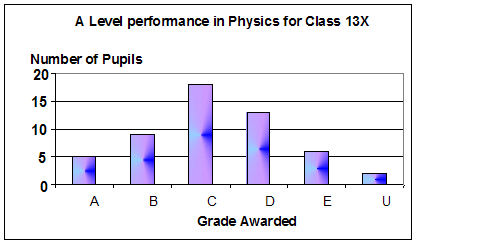
Bar charts help you to see how two or more separate entities (such as grades, years, different metals, animals, fish, countries) compare to each other. It is for displaying categoric variables.

.

Pie charts help you to see how the 'whole' is made up of various entities - to see the proportion of the contributions.

Line Graphs help you to see how two continuous variables relate to one another.

For science experiments you usually have to plot a 'best fit' line graph - a line graph can be a straight line or a curved line.

Graphs are a pictorial way of looking at data from a table. You can instantly see the 'trend' of your results and if you plot each set of data in a different colour on the same graph, you can also see the 'spread' the results and tell at a glance how precise your readings were.

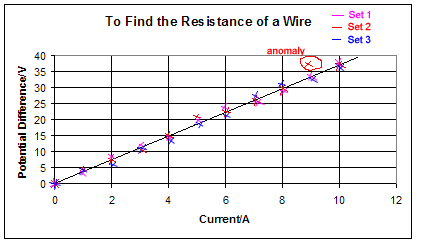
The graph above helps you to see the data gives you a general trend of direct proportionality and makes the ringed result stand out as an anomaly.

Plotting the spread of the results on the graph helps you to identify precision - not accuracy. Accuracy relates to how correct your results are - not your ability to get the same answer each time!

Sometimes examiners just want you to plot a single point for each measurement - then you need to plot your average result for that value. Such a graph does not indicate the precision of your results so it can be a good idea to include BOTH types of graph in a practical report - it gives you more to comment on in the results section.

When plotting a line graph you should:

Use a sharp pencil and a 12" ruler.

Draw the whole graph in pencil first and then when you are happy with it label the axes in ink, add a title in ink and if you wish go over your points in ink or in a fine-tipped felt or gel-ink pen.

Do NOT go over the line in ink.

You must:

choose an appropriate scale so that the:

(i) graph fills most of the page. It doesn't matter which way round you position the graph paper

(ii) divisions on the axes make it easy to plot the points accurately. Choose factors of 2 or 5 NOT 3 or 7, or any other awkward number.

Give the graph a title that explains what the experiment was about, not simply 'A graph of temperature against time'.... that can be gleaned from the labels on the axes.... something like 'Melting ice' explains what you were doing as you recorded temperature and time readings.

Put a key (explaining what each colour of line represents) if you choose to display more than one set of results on a single graph.

Label the axes with the physical quantity and the unit it was measured in. For example mass (kg)

Plot the points accurately and clearly. The best way to mark a point is to use a neat cross. If the line is then drawn so that it obliterates the point you can still see where it is.

Draw an appropriate best fit curved or straight line graph (NOT DOT-TO-DOT graphs) to fit the data, Your points are NOT perfect... your line gives an indication of the trend that they follow. Your line should be smooth... no 'bumps' or 'wiggles'!

A straight line should be drawn with a ruler, not freehand

A curved line should be drawn in a smooth 'swoop' through the points to indicate the general shape.... no 'bumps' or 'wiggles'!

Interpreting your graph

If your graph gives you a straight line it shows that the two physical quantities you plotted are proportional. If the straight line goes through the origin the graph indicates that they are directly proportional.... i.e. if you double one quantity the other will double too.

Any points that are well away from the line are called anomalies. They are probably due to experimental error. You should try to think of how these anomalies could have occurred or what you could do next time to avoid them happening.

The line you have drawn can be used to make predictions. You can draw a line parallel to one of the axes and then direct it towards the other axis after it has reached your graph line of best fit. This can be done from any value on either axis and allow you to predict what a pair of values in the experiment would probably be. In an exam always pencil in these lines to show the examiner how you reached your answer.

Finding the gradient of a straight line graph

The equation of a straight line graph is

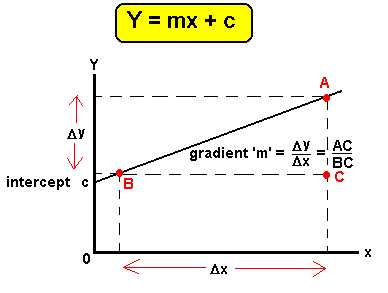
c is the intercept and

the gradient 'm' can be worked out from the change in Y (ΔY) values divided by the change in X (DX) values.

Here are the steps to follow when finding the gradient of your graph:

. Draw a LARGE (smallest side greater than 8cm) triangle, marking the vertices A,B and C and using dashed lines, as shown above.

Find what value the sides AC and BC represent by reading off the axes (don't forget their units!).

Write out the equation for the gradient EXACTLY as shown on the diagram above - do NOT miss out steps!

Calculate the gradient value

Write the value down in the same number of significant figures as it was possible to read from the axes.

Add the unit.