A cyclotron is an accelerator for charged particles.

A proton beam was used almost exclusively for physics research until the early sixties. Since that time there has been an increasing interest in using protons for medical radiotherapy.

A cyclotron is linked to other facilities such as spectrometers where work on the particles produced is done (see diagram of the Tex as A&M University Site below).

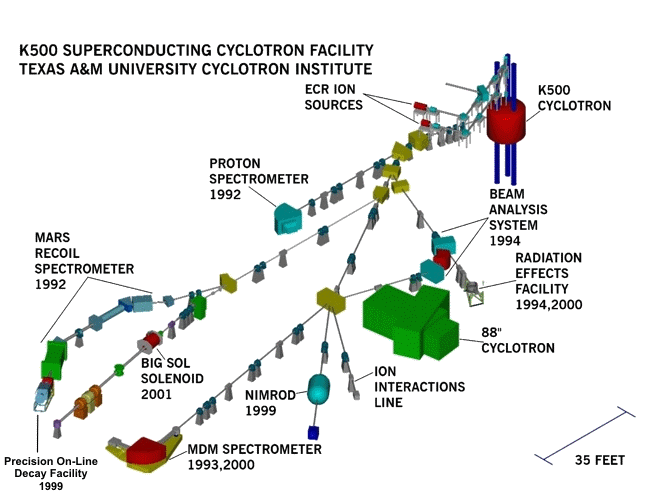
The K500 Cyclotron (illustrated above) is capable of supplying a variety of ion beams to the experiments at its Cyclotron Institute. Starting from the ECR ion sources, ion beams of the very lightest elements to ion beams of the very heaviest of elements can be accelerated by the cyclotron to high energies.

Beam energies for protons (ionized hydrogen) can range from 8 MeV to 70 MeV depending on the settings, while energies for uranium ions can range from 500 MeV (2MeV per nucleon) to 3.5 GeV (15 MeV per nucleon).

At the heart of the cyclotron is a superconducting magnet whose intense magnetic field holds the ions in orbits between its poles. The 50-kilogauss magnetic field is generated by 800 Amperes of electrical current carried by 5500 turns (25 miles) of niobium-titanium superconducting wire in a coil surrounded by 100 tons of steel (Faraday cage).

The acceleration of the ions is accomplished by intense, rapidly alternating electric fields generated by a 240-kilowatt radio-frequency system and impressed upon hollow copper structures called 'dees' located between the poles of the magnet. The generation, injection, acceleration and delivery to target of the ions takes place in high vacuum, while the path and focus of the beam outside the cyclotron is controlled by high-field, electromagnets.

Physically, a cyclotron weighs about from about 50 -120 tons (depending upon its date of manufacture and its capabilities - they are getting bigger and better all of the time!), and is located inside an inner vault with walls and doors of about 2 m concrete, to shield the surroundings from the nuclear radiation which is present when the machine runs. Fortunately, most of this radiation has a half-life of only seconds to minutes, so there are no long-term waste disposal problems.

The operating principle is illustrated below.

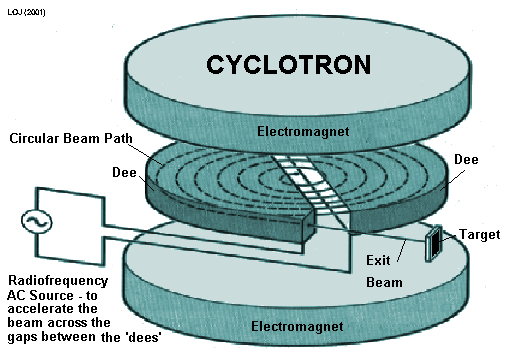
The ions (charged particles) are injected by an ion-source near the center of the machine (can't be seen clearly in this diagram). As they are charged and in a magnetic field they obey the formula:

F = qv x B

where

F is the force vector,

q is the charge,

v is the velocity vector and

B is the magnetic flux density vector.

Particles immediately start going in circular orbits. (The force is applied at right angles to their path - therefore they are forced into a circle)

For most of their orbit, the particles move within a hollow D-shaped metal electrode, called a "D" (or "dee").

Here they only experience the magnetic field, as they are screened from any electric field inside the cyclotron by what is known as Faraday Cage Effect.

The Faraday Cage Effect (named after its discoverer) means that the electric charge on a conductor sits on the outer surface of it. Therefore, no electrostatic field is present within the conductor. Only when the particles move in the small gap between the dees, they are influenced by an electric field applied from one dee to the other.

We will then get an acceleration of the particles, according to:

F = qE

where

F is the force vector,

q is the charge and

E is the electric field intensity vector.

There will only be maximum acceleration if the electric force has the same direction as the velocity. Therefore, the electric field must change its direction while the particles are screened inside the dees so that the electric field (the dee-voltage is typically ~ 100 kV) can be applied in one direction as it leaves the one 'dee' and be in the opposite direction by the time it leaves the other 'dee' half a cycle later. This means that the electric field has to oscillate with a frequency corresponding to the particles' revolution frequency. In practice his frequency is in the radiofrequency range (typically in the range 12 - 24 MHz, in the middle of the shortwave band).

Now, the magnetic force is equal to the centripetal force of the circular movement (it is what makes it move in a circle!), so we get the cyclotron equation from equating the two:

Centripital force = mv²/r

Magnetic force = Bvq

 

\ mv²/r = Bvq

Cancelling a ' v ' and rearranging we have

where r is the radius of the particle orbit.

As we can see from this, as the particles increase their velocity (because they have been accelerated between the 'dees' their radius will increase as radius is proportional to their velocity.

This is repeated until they after typically a few hundred orbits reach the energy desired. They can then be extracted by a new electric field combined with a magnetic field, and be transported through a beam tube to the point where they are to be used to initiate nuclear reactions.

The beam line is a straight steel tube of diameter about 10cm, in which vacuum of about 0.001Pa is kept, and where the beam runs from its source (in this case the cyclotron) to the area where it is used for bombarding target matter.

The K500 Cyclotron with its upper steel pole cap raised for maintenance. The exit beam line is at lower left.

Extension work - especially relevant to those doing relativity in their option!

Notice that above equation requires the mass 'm' to be constant for 'v' to be directly proportional to r. Relativistically, the mass is not constant, but increases with v. This does not matter for low speeds but is highly relavant in this context, since for instance 35 MeV protons have a velocity of about 0.3c.

Medical uses of the Cyclotron - the manufacture of radioactive materials

Some radioactive elements, such as radium, are found in nature, but most radioactive materials are produced commercially in nuclear reactors or cyclotrons. With nuclear reactors and cyclotrons, it is possible to make useful amounts of radioactive material safely and at low cost - often on site at a large hospital - important when the radioisotope has a short half life.

A cyclotron uses electric current to accelerate charged atomic particles such as protons, which strike the non-radioactive "target" material, turning it into a radioactive isotope.

When the non-radioactive "target" element cobalt is struck by neutrons in a reactor, it is transformed into a radioisotope (cobalt-60) which is used to treat cancer and sterilize medical and consumer products.

The difference between cyclotron and reactor manufacturing is that usually only one type of radionuclide can be produced at a time in a cyclotron, while a reactor can produce many different radionuclides simultaneously.

If radionuclides are produced commercially, they are packaged and safely shipped to users throughout the country, including hospitals, laboratories, universities and manufacturing plants, but large hospital facilities may well have a cyclotron on site if they have equipment such as a PET scanner..